Nonlinear analysis of reinforced concrete shells and the compressive membrane action on bridge deck slabs

Análise não-linear de cascas de concreto armado e o efeito de membrana comprimida em lajes de tabuleiro de pontes

Abstracts

Abstract  There are reinforced concrete problems where it is essential to analyze the reinforcement stresses at the crack, such as verifying the Fatigue Limit State in bridge deck slabs and crack opening in water tanks. In view of this, this work presents a numerical model called Cracked Shell Model (CSM), which enables the determination of stresses in the orthogonal reinforcement of concrete shell elements. The CSM uses the layered method, where the thickness of the shell element is discretized into concrete layers subjected to a plane stress state. The reinforcements are evaluated using the Cracked Membrane Model (CMM), which considers the compression softening of concrete and the tension stiffening effects via the Tension Chord Model (TCM). The validation of the CSM was carried out with experimental and numerical shell data available in the literature. It has been shown to be capable of predicting the complete behavior of reinforced concrete shell elements, with good accuracy and low computational cost. Additionally, a study was conducted on the Compressive Membrane Action (CMA) on bridge deck slabs, which tends to increase the load capacity of these slabs. With this study it can be observed that the CSM, despite the good performance presented in the validation phase, as it is based on Kirchhoff thin plate theory (usual practice in many projects) failed to capture this effect, underestimating the ultimate load by an average of 44% in relation to experimental tests. This article aims to contribute to developing numerical models of reinforced concrete shells, in problems where the maximum reinforcement stress is essential.

Keywords:
shells; reinforced concrete; serviceability behavior; reinforcement stresses at the crack; compressive membrane action

Resumo  Existem problemas de cascas de concreto onde é imprescindível analisar as tensões das armaduras na fissura, como a verificação do Estado Limite Último de fadiga em lajes de tabuleiros de pontes e abertura de fissuras em reservatório de água. Em vista disso, este trabalho apresenta o modelo numérico denominado Modelo de Casca Fissurada (Cracked Shell Model – CSM), que possibilita determinar as tensões nas armaduras ortogonais de elementos de casca de concreto armado. O CSM utiliza o método lamelar, onde a espessura do elemento de casca é discretizada em lamelas de concreto sujeitas a um estado plano de tensões. As armaduras são avaliadas a partir do Modelo de Membrana Fissurada (Cracked Membrane Model - CMM), que considera o amolecimento do concreto à compressão e o efeito de enrijecimento à tração via Modelo de Banzo Tracionado (Tension Chord Model - TCM). A validação do CSM foi realizada com dados experimentais e numéricos de cascas disponíveis na literatura, no qual o modelo mostrou ser capaz de prever o comportamento completo de elementos de casca de concreto armado, com boa precisão e baixo custo computacional. Adicionalmente, foi conduzido um estudo sobre o Efeito de Membrana Comprimida em lajes de tabuleiro de pontes, o qual tende a aumentar a capacidade resistente dessas lajes. Com este estudo pode-se observar que o CSM, apesar do bom desempenho apresentado na fase de validação, por está baseado na teoria de placas finas de Kirchhoff (prática usual em muitos projetos) não conseguiu capturar esse efeito, subestimando a carga última, em média em 44%, em relação à ensaios experimentais. Esse artigo visa contribuir para o desenvolvimento de modelos numéricos de cascas de concreto armado, em problemas onde a tensão máxima da armadura é fundamental.

Palavras-chave:
cascas; concreto armado; comportamento em serviço; tensões na armadura na fissura; efeito de membrana comprimida

1 INTRODUCTION

Reinforced concrete (RC) shell elements are present in several civil engineering constructions, such as bridge decks and water tanks. These elements are characterized by two dimensions being much larger than the third one. In addition, they can be subjected to both tangential and normal forces. In bridges, due to the moving load condition, which implies a considerable stress fluctuation in the structure, the shell orthogonal reinforcement is subject to the fatigue phenomenon. In cyclic loading tests carried out by Eshwarappa and Gangolu [¹], it was found that it is at the crack where the greatest stress fluctuations occur, since the reinforcement tends to fail in this location. Therefore, it is essential to know the reinforcement stresses in local terms (i.e., at the crack) to evaluate the Fatigue Limit State. ABNT NBR 6118 [²] presents detailed criteria for the design of structural elements subject to the fatigue phenomenon. On the other hand, according to the American Association of State Highway and Transportation Officials - AASTHO [³], this verification is unnecessary for multigirder bridges whose spacing between beam axes is not greater than 4.1 meters. The difference in the approach to the problem of fatigue by the two aforementioned standards and the reasons that justify it, motivated this study.

Some important contributions to the treatment of the RC shell model considering the behavior of steel at the crack were given by Kvam [⁴], Pimentel [⁵] and Spathelf [⁶]. They used numerical models based on the discretization of shell thickness into concrete layers, subjected to a plane stress state, and reinforcement layers evaluated as RC membranes (Cracked Membrane Model - CMM) [⁷]. Some basic aspects of this model and the contributions of the referred authors are discussed following. The CMM establishes the equilibrium of the element at the crack, where the crack faces are assumed to be stress-free and able to rotate. Furthermore, it considers the compression softening of concrete and the tension stiffening effects based on the Tension Chord Model (TCM) idealized by Marti et al. [⁸]. In the work of Spathelf [⁶], the original version of the CMM is implemented for fatigue problems in slabs, considering the tangent constitutive matrices of the materials. Kvam [⁴] applied the iteration method presented by Overli and Sorensen [⁹], starting from a preliminary elastic and linear analysis of the structure, to estimate the crack opening in RC shells. Differently from the previous ones, Pimentel [⁵] presents a code implemented via finite elements and the layers are evaluated according to the CMM-F model (where the cracks are fixed and interlocked, capable of transferring shear and normal stresses). These works demonstrated the feasibility of using layered and CMM models to evaluate RC shells.

Independently of the consistency of a model, it is essential to consider the efficiency of the numerical procedure in its computational implementation. In nonlinear models, in which iterative methods are used to solve the problem, the definition of the initial estimative can considerably affect this efficiency, Fernandes [¹⁰], Fernandes et al. [¹¹]. Depending on the estimative, the model may provide unacceptable results; or even not converge to any solution. The same efficiency can also be impacted by the stiffness matrix adopted. In this regard, problems that are not continually differentiable, depending on this matrix, may present convergence difficulties, Silva [¹²]. The computational processing time demonstrated by the model, therefore, becomes an important measure to verify these aspects.

Based on the discussions above, in the first part of this article, the nonlinear numerical model for RC shell analysis, Cracked Shell Model (CSM), is presented, which is capable of evaluating the behavior of sections of RC shells up to rupture, including calculating the stresses in the reinforcement at the crack. The shell thickness is discretized in layers, modeled via CMM. The Newton-Raphson method is adopted to solve a nonlinear problem, considering the material secant stiffness. The model bases and the differences between this implementation and others in the literature, previously mentioned are detailed. The numerical code was validated with experimental data of the literature. Good prediction of the results compared to the experimental ones can be observed, including in some cases, better than the other numerical solutions compared. Regarding the computational cost, the processing time varied between 8 and 15 seconds, using a computer with an Intel Core i5-5200U CPU @ 2.20GHz processor, with analyses involving torsion being the most costly. The Kirchhoff thin plate theory was considered as the estimate solution, for the first iteration.

In the second part of the article, a case study is presented, where, using the CSM, the influence of the Compressive Membrane Action (CMA) on bridge deck slabs is evaluated. This effect results from the concrete cracking and the slab horizontal translational restraint of the slab, enabling an increase in its strength capacity. Experimental results of a RC slab are compared with the ultimate load provided by the CSM. It is considered the ratio between bending and torsional moments in the slab, obtained via a linear elastic analysis. As the CSM is based on Kirchhoff thin plate theory, it was unable to capture the beneficial effect of the CMA, resulting in a rupture load on average 44% lower than that obtained in tests. The main purpose of this comparison is precisely to highlight this limitation.

This article aims to contribute to developing numerical models for analyzing RC shells in situations where knowledge of the maximum reinforcement stresses is essential. It also discusses the influence of CMA on the behavior of bridge deck slabs, highlighting the inability of usual models, even those with a certain complexity and experimental validation, to accurately portray this effect.

2 CONSTITUTIVE MODELS

For numerical analysis of reinforced concrete structures, it is necessary to establish constitutive models that characterize the behavior of the materials. In this study, the membrane constitutive models are divided into cracked or uncracked concrete models. These models are later applied in shell analysis. The formulation of constitutive models, including the CMM and TCM, is illustrated in Figure 1. The nomenclature of the unknowns is described in Table 1.

Figure 1
Material constitutive models.

Table 1
Nomenclature.

Notation	Description	Notation	Description
$A_{load}$	load area	$N_x$, $N_z$, $N_{xz}$	forces in the 𝑥 – 𝑧 plane
$A_{s,j}$, $A_{sx,j}$, $A_{sz,j}$	steel area of layer 𝑗 in the 𝑥 and 𝑧 directions	$P_u^{CSM}$, $P_u^{exp}$	failure load obtained via CSM and experiment
$c_{nom}$	nominal concrete cover to the reinforcement	$q_{SAP}$	load applied for SAP2000 analysis
${erro}_{n+1}$	auxiliary parameter of the convergence criterion in iteration n + 1	$s_{rm}$	crack spacing
$E_c$, $E_s$	concrete and steel Young modulus	$s_{rmx}$, $s_{rmz}$	crack spacing in the $x$ and $z$ directions
$E_{c1,sec}$, $E_{c3,sec}$	concrete secant modulus in the 1 and 3 directions	$s_{rm0}$	maximum crack spacing
$E_{c13,sec}$	concrete secant modulus in the 𝑥 – 𝑧 plane	$s_{rmx0}$, $s_{rmz0}$	maximum crack spacing in the $x$ and $z$ directions
$E_{sh}$	steel hardening modulus	$t$	scaling factor of normalized vector
$f_c$	uniaxial compressive concrete strength	$tol$	tolerance admitted for the convergence criterion
$f_p$	peak compressive stress	$V_x$, $V_z$	shear force in x and z directions
$f_{cr}$	concrete tensile strength	$u$, $v$, $w$	displacements in the u, v and w directions
$f_{sy}$	steel yield stress	$x_1,$ $x_2$	first and second transfer length
$f_{su}$, $f_{sux}$, $f_{suz}$	steel ultimate stress in x and z directions	$y_{s,j}$, $y_{sT}$, $y_{sB}$	lever arm of the reinforcement layer $j$, top and bottom shell
$h$	shell height	$y_i$, $y_{i+1}$	top and bottom position of the concrete lamella 𝑖
$k$, $l$	layers number of concrete and steel	$\left\{F_{ext}\right\}$, $\left\{F_{int}\right\}$	external and internal forces vector
$M_x$, $M_z$, $M_{xz}$	moments in the 𝑥 – 𝑧 plane	$\left[C\right]$	position matrix
$M_r^{CSM}$, $M_u^{CSM}$	cracking and ultimate moment of the CSM	$\left[D_{13}^c\right]$	concrete secant constitutive matrix in plane $1-3$
$M_r^{exp}$, $M_u^{exp}$	cracking and ultimate moment of the experiment	$\left[D_{xz}^c\right]$, ${\left[D_{xz}^c\right]}_i$	concrete secant constitutive matrix in plane $x-z$ in the lamella $i$
$M_x^{CSM}$	bending moment in the 𝑥-direction of the CSM	$\left[D_{\mathrm{}}^s\right]$	steel secant constitutive matrix
$M_x^{SAP}$, $M_z^{SAP}$, $M_{xz}^{SAP}$	moments in the 𝑥 – 𝑧 plane obtained via SAP2000	$\left[D_{xz}^s\right]$, ${\left[D_{xz}^s\right]}_j$	steel constitutive matrix in the 𝑥 − 𝑧 plane of the 𝑗 layer
${\left[K_{shell}^c\right]}_i$, ${\left[K_{shell}^s\right]}_j$	concrete and steel stiffness matrix in layers $i$ and $j$	${\kappa }_x$, ${\kappa }_z$, ${\kappa }_{xz}={\kappa }_{zx}$	curvatures of the $x-z$ plane
$\left[D_{xz}\right]$	constitutive matrix of the material in the 𝑥 − 𝑧-plane	$\lambda$	coefficient regulating crack spacing [0.5 ≤ λ ≤ 1.0]
$\left[K_{shell}\right]$	shell stiffness matrix	$\nu$	Poisson ratio for concrete
$\left[K_{shell}^c\right],\mathrm{}\left[K_{shell}^s\right]$	concrete and steel stiffness matrix	$\rho$	geometrical reinforcement ratio
$\left\{R\right\}$	force unbalance vector	${\sigma }_{c1}$, ${\sigma }_{c3}$	concrete principal stresses
$\left[T\right]$	transformation matrix	${\sigma }_c$, ${\sigma }_{cx,i}$, ${\sigma }_{cz,i}$, ${\tau }_{cxz,i}$	concrete stresses in the $x-z$ plane of layer $i$
${\gamma }_{yx}$, ${\gamma }_{yz}$	shear strains in the y − x and y − 𝑧 planes	${\sigma }_s$, ${\sigma }_{sx,j}$, ${\sigma }_{sz,j}$	steel stresses in the $x$ and $z$ direction of layer $j$
${∆y}_{sT}$, ${∆y}_{sB}$	thickness of bottom and top cover elements	${\sigma }_{sm}$, ${\sigma }_{smin}$	steel average stress and minimum steel stress
${\epsilon }_1$, ${\epsilon }_3$	initial average strain in the $1$ and 3 directions	${\sigma }_{sr}$, ${\sigma }_{sr1},$${\sigma }_{sr2},$${\sigma }_{sr3}$	steel stress at the crack in regimes 1, 2 and 3
${\epsilon }_{c3,sup},\mathrm{}{\epsilon }_{c3,inf}$	minimum principal strain of the top and bottom layer	${\sigma }_{sx},$ ${\sigma }_{sz}$	steel stresses in x and z directions
${\epsilon }_{co}$	concrete strain corresponding to the peak stress	${\sigma }_x$, ${\sigma }_z,$${\tau }_{xz}$	external stresses in the 𝑥 – 𝑧 plane
${\epsilon }_{cr}$	cracking strain	${\tau }_{b0}$, ${\tau }_{b1}$	levels of bond stress describing bond stress-slip relationship
${\epsilon }_{mx}$, ${\epsilon }_{mz}$, ${\gamma }_{mxz}={\gamma }_{mzx}$	average surface strains of the 𝑥 − 𝑧 plane	${\phi }_x$, ${\phi }_z$	rotation of straight fiber in x and z directions
${\epsilon }_{sm}$, ${\epsilon }_{smx}$, ${\epsilon }_{smz}$	steel average strain in the $x$ and $z$ directions	$\left\{\Delta {\epsilon }_t\right\}$	increment vector of strain and curvatures
${\epsilon }_{sy},$ ${\epsilon }_{su}$	steel yielding and ultimate strain	$\left\{{\epsilon }_{xz}\right\}$	strain vector in the 𝑥 − 𝑧 plane
${\epsilon }_x,$${\epsilon }_z$, ${\gamma }_{xz}$	strains in plane $x-z$	$\left\{{\epsilon }_t\right\}$	strains and curvatures vector
$\theta$, ${\theta }_r$	angle of the coordinate system in relation to the $x-z$ plane and crack angle	${\mathrm{ø}}_s$, ${\mathrm{ø}}_{sxT}$, ${\mathrm{ø}}_{sxB}$	bar diameter in 𝑥 direction of top and bottom layer
${\kappa }_p$	maximum principal bending curvature

For uncracked RC membranes, when the maximum principal strain ${\epsilon }_1$ is lower than the cracking strain ${\epsilon }_{cr}$, the concrete under tension exhibits linear elastic behavior until it reaches its tensile strength $f_{cr}$. In compression, the behavior of concrete in the pre-peak range can be approximated by a quadratic function dependent on the concrete peak stress $f_p$, the peak strain ${\epsilon }_{co}$ and the compression principal strain ${\epsilon }_3$. For membrane elements subjected to a biaxial plane state of compression and tension stresses, the peak stress $f_p$ is adjusted to account for concrete compression softening. This occurs due to the principal tensile strain ${\epsilon }_1$ perpendicular to the concrete compression direction, which leads to a reduction in compressive strength. To account for this effect in the quadratic function, a correction is applied only in the strength (stress softening), based on the principal tensile strain ${\epsilon }_1$ [⁷]. For the steel reinforcement, in both compression and tensile, it is considered a bilinear model. The dowel effect is disregarded.

For cracked RC membranes, the Cracked Membrane Model (CMM) is used when ${\epsilon }_1$>${\epsilon }_{cr}$, as shown in Figure 1. This model is based on the Modified Compression Field Theory (MCFT) [¹³] and the Tension Chord Model (TCM) [⁸]. In CMM, equilibrium is defined at the crack, which is stress-free, capable of rotation, and oriented perpendicular to the principal tensile strain direction. The compressive behavior of concrete follows the same quadratic function as in the constitutive model for uncracked membranes. If needed, the peak strength is adjusted to account for concrete compression softening. The reinforcement in tension behavior is evaluated using the TCM, which incorporates the tension stiffening effect. This effect arises from the bond between the concrete and the steel, and assuming that the uncracked concrete between cracks, surrounding the steel bar, provides resistance to tension. In TCM, the bond shear stress between concrete and reinforcement is modeled as exhibiting perfectly plastic rigid behavior with two levels [¹⁴]. At the first level, when the reinforcement is not yielded, the bond shear stress is twice the concrete cracking strength $f_{cr}$, i.e., ${\tau }_{b0}=2f_{cr}$. At the second level (yielded reinforcement), this stress reduces to ${\tau }_{b1}=f_{cr}$ [¹⁴]. This bond model allows for the analytical calculation of steel stresses at the crack ${\sigma }_{sr}$ as a function of the average strain ${\epsilon }_{sm}$. Consequently, the steel stress at the crack ${\sigma }_{sr}$ can fall into three distinct regimes: steel not yielded, steel partially yielded, and steel fully yielded, as illustrated in Figure 1. In cases where there is a slip between the concrete and the steel, typically near the crack under low load levels, the steel stress at the crack can be recalculated following the method outlined by Seelhofer [¹⁵]. Further details on the implementation of the CMM and TCM can be found in references by Fernandes [¹⁰] and Fernandes et al. [¹¹].

3 THIN SHELLS

3.1 Reinforced concrete shells

The RC shell analysis procedure implemented uses the layered method based on the works of Barrales [¹⁶], Zhang et al. [¹⁷], Spathelf [⁶], and Silva [¹²], as illustrated in Figure 2. According to this approach, the concrete is discretized into $l$ layers and the reinforcement into $k$ layers, both subject to a stress plane state. The contributions of each material to the equilibrium results in the shell element behavior.

Figure 2
Procedure for cracked shell solution.

For each concrete layer, the respective constitutive relations of the material are adopted, and the deformation field is evaluated in the layer mid-plane, being uniform within each layer. The orthogonal reinforcements next to the top and bottom surfaces of the shell are considered embedded into fictitious membrane elements, to consider the tension stiffening effect caused by the concrete around the bar. Therefore, to evaluate the behavior of the reinforcement, the deformation field is evaluated in the center of the fictitious membrane element [⁶].

The thickness of the respective membranes containing the reinforcement is indicated by ${∆y}_{sT}$ and ${∆y}_{sB}$ and is determined by Equation 1 [⁶]. The expressions are functions of the nominal concrete cover $c_{nom}$ and the diameter of the bar parallel to the $x$-axis, where ${ø}_{sxT}$ corresponds to the top membrane element and ${ø}_{sxB}$ to the bottom membrane element. It is considered that the bars in the $x$-direction are those close to the surface, as shown in Figure 2.

${∆y}_{sT}=2(c_{nom}+{\mathrm{ø}}_{sxT});\mathrm{}{∆y}_{sB}=2(c_{nom}+{\mathrm{ø}}_{sxB})$ (1)

3.2 Deformation compatibility

For the bending behavior of the shell element, the hypotheses of Kirchhoff thin plates theory are considered, i.e., small deflections, vertical displacements occurring only in the $y$-direction, and straight lines initially normal to the mid-plane remaining straight and normal to this surface after deformations. A consequence of this hypothesis is that the shear strains ${\gamma }_{yx}$ and ${\gamma }_{yz}$ are despised. Based on these hypotheses and considering the deformed configuration in Figure 3b, the deformations of each fiber are calculated as a function of the curvatures caused by the bending and torsion moments.

Figure 3
Shell element and structure flexural behavior adapted from Leitão and Castro [¹⁸].

The compatibility equations for the deformations of the shell element result from the axial and shear deformations in the mid-plane (${\epsilon }_{mx}$, ${\epsilon }_{mz}$ and ${\gamma }_{mzx}={\gamma }_{mxz}$), originating from the membrane forces, and the deformations arising from the curvatures (${\kappa }_x$, ${\kappa }_z$ e ${\kappa }_{zx}={\kappa }_{xz}$) generated by bending and torsion moments. Considering that the mid-plane of the shell coincides with the $x-z$ plane, the equations Equation 2, Equation 3 and Equation 4 express the compatibility equations of a fiber distant $y$ from this plane, see Figure 3.

${\epsilon }_x\left(y\right)={\epsilon }_{mx}+y{\kappa }_x$ (2)

${\epsilon }_z\left(y\right)={\epsilon }_{mz}+y{\kappa }_z$ (3)

${\gamma }_{xz}\left(y\right)={\gamma }_{mxz}+2y{\kappa }_{xz}$ (4)

The terms ${\epsilon }_{mx}$, ${\epsilon }_{mz}$, ${\gamma }_{mxz}$, ${\kappa }_x$, ${\kappa }_z$ and ${\kappa }_{xz}$ are constants for each shell section, so the above equations can be rewritten as in Equation 5. The vector of strains and curvatures $\left\{{\epsilon }_t\right\}$ is defined by Equation 6, and the fiber position matrix $\left[C\right]$ is defined by Equation 7.

$\left\{{\epsilon }_{xz}\right\}=\left[C\right]\left\{{\epsilon }_t\right\}$ (5)

$\left\{{\epsilon }_t\right\}={\{{\epsilon }_{mx},\mathrm{}{\epsilon }_{mz},\mathrm{}{\gamma }_{mxz},\mathrm{}{\kappa }_x,\mathrm{}{\kappa }_z,2{\kappa }_{xz}\}}^T$ (6)

$\left[C\right]=\left[\begin{array}{cccccc}1& 0& 0& y& 0& 0\\ 0& 1& 0& 0& y& 0\\ 0& 0& 1& 0& 0& y\end{array}\right]$ (7)

3.3 Equilibrium equations

From a statics point of view, the sum of the forces developed by the internal stresses of each fiber of the structure must be equal to the external forces applied for the structure to be considered in equilibrium, i.e., the unbalance vector $\left\{R\left({\epsilon }_t\right)\right\}$, shown in Equation 8, must be zero. The applied membrane forces $N_x$, $N_z$, $N_{xz}=N_{zx}$, and the bending and torsional moments $M_x$, $M_z$, $M_{xz}=M_{zx}$, are expressed per unit length. The term $y$ is the coordinate of the layer axis.

$\left\{R\left({\epsilon }_t\right)\right\}=\left\{F_{ext}\right\}-\left\{F_{int}\left({\epsilon }_t\right)\right\}=\left\{\begin{array}{c}{N}_x\\ N_z\\ N_{xz}\\ M_x\\ M_z\\ M_{xz}\end{array}\right\}-{\int }_{-h/2}^{h/2}\left\{\begin{array}{c}{\sigma }_x\mathrm{}\\ {\sigma }_z\\ {\tau }_{xz}\\ y{\sigma }_x\\ y{\sigma }_z\\ y{\tau }_{xz}\end{array}\right\}dy$ (8)

Considering the layered method [⁶], [¹²], [¹⁶], [¹⁷], the internal force vector can be calculated numerically as the sum of the contributions of each concrete and reinforcement layer, as shown in Equation 9. This vector is composed of nonlinear equations, and the stresses depend on the strain-curvature vector {${\epsilon }_t$}.

$\left\{F_{int}\left({\epsilon }_t\right)\right\}=\sum _{i=1}^l\left\{\begin{array}{c}{\sigma }_{cx,i}(y_i-y_{i+1})\\ {\sigma }_{cz,i}(y_i-y_{i+1})\\ {\tau }_{cxz,i}(y_i-y_{i+1})\\ \frac{1}{2}{\sigma }_{cx,i}({y_i}^2-{y_{i+1}}^2)\\ \frac{1}{2}{\sigma }_{cz,i}({y_i}^2-{y_{i+1}}^2)\\ \frac{1}{2}{\tau }_{cxz,i}({y_i}^2-{y_{i+1}}^2)\end{array}\right\}+\sum _{j=1}^k\left\{\begin{array}{c}{\sigma }_{sx,j}{A}_{sx,j}\\ {\sigma }_{sz,j}{A}_{sz,j}\\ 0\\ {\sigma }_{sx,j}{A}_{sx,j}{y}_{s,j}\\ {\sigma }_{sz,j}{A}_{sz,j}{y}_{s,j}\\ 0\end{array}\right\}$ (9)

3.4 Secant constitutive matrices

The secant constitutive matrix of concrete adopted for the numerical model presented in this work is the same as that proposed by Kvam [⁴], which includes the Poisson effect in its formulation. In contrast, authors such as Spathelf [⁶] and Pimentel [⁵] use tangent constitutive matrices. The secant constitutive matrix, as shown in Equation 10, is calculated for each layer $i$ in the $1-3$ principal system. The secant strain modules $E_{c1,sec}$, $E_{c3,sec}$ and $E_{c13,sec}$ are determined according to Equation 11.

$\left[D_{13}^c\right]=\frac{1}{1-{\nu }^2}\left[\begin{array}{ccc}{E}_{c1,sec}& {\nu E}_{c13,sec}& 0\\ {\nu E}_{c13,sec}& E_{c3,sec}& 0\\ 0& 0& {\frac{(1-\nu )}{2}E}_{c13,sec}\end{array}\right]$ (10)

$E_{c1,sec}=\frac{{\sigma }_{c1}}{{\epsilon }_{c1}};\mathrm{}{E}_{c3,sec}=\frac{{\sigma }_{c3}}{{\epsilon }_{c3}};\mathrm{}{E}_{c13,sec}=\frac{E_{c1,sec}+E_{c3,sec}}{2}$ (11)

The transformation of the matrix $\left[D_{13}^c\right]$ to the $x-z$ global axis system, resulting in the matrix $\left[D_{xz}^c\right]$ Equation 13, is carried out using the transformation matrix $\left[T\right]$ defined in Equation 12. The angle $\theta$, in this case, corresponds the principal strains direction. In Spathelf [⁶], the concrete constitutive matrix is summed to an additional rotational stiffness matrix. According to the author, in the event of a change in the crack direction in subsequent load steps, this matrix aims to enhance numerical convergence. In this work, however, this additional matrix is ​​not applied.

$\left[T\right(\theta \left)\right]=\left[\begin{array}{ccc}{cos}^2\left(\theta \right)& {sen}^2\left(\theta \right)& cos\left(\theta \right)sen\left(\theta \right)\\ {sen}^2\left(\theta \right)& {cos}^2\left(\theta \right)& -cos\left(\theta \right)sen\left(\theta \right)\\ -2cos\left(\theta \right)sen\left(\theta \right)& 2cos\left(\theta \right)sen\left(\theta \right)& {cos}^2\left(\theta \right)-{sen}^2\left(\theta \right)\end{array}\right]$ (12)

$\left[D_{xz}^c\right]={\left[T\right({\theta }_{\mathrm{}}\left)\right]}^T\left[D_{c13}\right]\left[T\right({\theta }_{\mathrm{}}\left)\right]$ (13)

In turn, the secant constitutive matrix of the reinforcement layer $\left[D_{}^s\right]$ is calculated using Equation 14. In Pimentel [⁵] and Spathelf [⁶], the term $E_s$ is obtained by differentiating ${\sigma }_s$ with respect to ${\epsilon }_{sm}$.

$\left[D_{\mathrm{}}^s\right]=\left[\begin{array}{ccc}{E}_s& 0& 0\\ 0& 0& 0\\ 0& 0& 0\end{array}\right]\mathrm{}{E}_s=\frac{{\sigma }_s}{{\epsilon }_{sm}}$ (14)

The matrix $\left[D_{}^s\right]$ is transformed to the $x-z$ global axis system $\left[D_{xz}^s\right]$ using the transformation matrix $\left[T\right(\theta \left)\right]$, as shown in Equation 15. Here, the angle $\theta$ corresponds the reinforcement orientation. Since the reinforcement orientation aligns with the global axes, this direction can take values ​​of 0º or 90º.

$\left[D_{xz}^s\right]={\left[T\right(\theta \left)\right]}^T\left[D_s\right]\left[T\right(\theta \left)\right]$ (15)

3.5 Stiffness matrix

From the equilibrium equation in Equation 8, by expressing the internal force vector in terms of the fiber position matrix $\left[C\right]$ Equation 7, and the material constitutive matrix $\left[D_{xz}\right]$, the shell problem can be formulated as shown in Equation 16, according to Vasilescu [¹⁹] apud Silva [¹²]. Since the strain-curvature vector $\left\{{\epsilon }_t\right\}$ is constant for any point on the shell, it remains external to the integration term. The resulting integration term is the shell stiffness matrix $\left[K_{shell}\right]$.

$\left\{F_{ext}\right\}={\int }_{-h/2}^{h/2}{\left[C\right]}^T\left[D_{xz}\right]\left[C\right]dy\left\{{\epsilon }_t\right\}=\left[K_{shell}\right]\left\{{\epsilon }_t\right\}$ (16)

Performing the multiplication of the integral terms and organizing them, the shell stiffness matrix results in the Equation 17.

$\left[K_{shell}\right]={\int }_{-h/2}^{h/2}\left[\begin{array}{cc}\left[D_{xz}\right]& y\left[D_{xz}\right]\\ y\left[D_{xz}\right]& y^2\left[D_{xz}\right]\end{array}\right]dy\mathrm{}$ (17)

Considering the layered method discussed in section 3.1, the integral Equation 17 can be numerically solved as the sum of the individual contributions from each concrete and reinforcement layer, $\left[K_{shell}\right]=\left[K_{shell}^c\right]+\left[K_{shell}^s\right]$. The contribution of the concrete layers to the shell stiffness $\left[K_{shell}^c\right]$, is defined by Equation 18.

$\left[K_{shell}^c\right]\mathrm{}=\sum _{i=1}^l\left[\begin{array}{cc}{y}_i-y_{i+1}{\left[D_{xz}^c\right]}_i& \frac{{y_i}^2-{y_{i+1}}^2}{2}{\left[D_{xz}^c\right]}_i\\ \frac{{y_i}^2-{y_{i+1}}^2}{2}{\left[D_{xz}^c\right]}_i& \frac{{y_i}^3-{y_{i+1}}^3}{3}{\left[D_{xz}^c\right]}_i\end{array}\right]$ (18)

In turn, the contribution of the steel layers to the shell stiffness, $\left[K_{shell}^s\right]$, is expressed in Equation 19, where $A_{s,j}$ represents the steel area of ​​layer $j$. The lever arm, $y_{s,j}$, corresponds to the distance from the shell mid-plane to the center of the fictitious membrane element into which the reinforcement is inserted. Therefore, the lever arm equals $y_{sB}$, for the bottom orthogonal reinforcement, and $y_{sT}$ for the top orthogonal reinforcement, both calculated by Equation 20 [⁶].

$\left[K_{shell}^s\right]=\sum _{j=1}^k\left[\begin{array}{cc}{A}_{s,j}{\left[D_{xz}^s\right]}_j& A_{s.j}{y}_{s,j}{\left[D_{xz}^s\right]}_j\\ A_{s,j}{y}_{s,j}{\left[D_{xz}^s\right]}_j& A_{s,j}{y_{s,j}}^2{\left[D_{xz}^s\right]}_j\end{array}\right]$ (19)

$y_{sT}=\frac{{∆y}_{sT}-h}{2};\mathrm{}{y}_{sB}=\frac{h-{∆y}_{sB}}{2}$ (20)

3.6 Procedure to solve the nonlinear equations system

According to Silva [¹²], the nonlinear shell problem in study can be solved using the equation Equation 21, where the variable to be determined is the curvature-strain vector $\left\{{\epsilon }_t\right\}$.

$\left\{R({\epsilon }_t+\mathrm{\Delta }{\epsilon }_t)\right\}=\left\{R\left({\epsilon }_t\right)\right\}-\left[K_{shell}\left({\epsilon }_t\right)\right]\left\{\mathrm{\Delta }{\epsilon }_t\right\}$ (21)

The solution of Equation 21 requires that the force unbalance vector $\left\{R({\epsilon }_t+\mathrm{\Delta }{\epsilon }_t)\right\}$ equals zero. This equation can be solved through successive iterations using the Newton-Raphson numerical method. The strain-curvature increment vector $\left\{\mathrm{\Delta }{\epsilon }_t\right\}$ is calculated by Equation 22, and the new strain vector ${\left\{{\epsilon }_t\right\}}_{n+1}$ to be evaluated is given by Equation 23.

${\left\{\mathrm{\Delta }{\epsilon }_t\right\}}_{n+1}={\left[K_{shell}\left({{\epsilon }_t}_n\right)\right]}^{-1}(\left\{F_{ext}\right\}-\left\{F_{int}\left({{\epsilon }_t}_n\right)\right\})$ (22)

${\left\{{\epsilon }_t\right\}}_{n+1}={\left\{{\epsilon }_t\right\}}_n+{\left\{\mathrm{\Delta }{\epsilon }_t\right\}}_{n+1}$ (23)

The convergence criterion is determined by the tolerance $tol$ set for the analysis. When the parameter ${error}_{n+1}$, calculated using Equation 24, is less than or equal to the defined tolerance, it indicates that the nonlinear equations system has converged to a solution. Consequently, the iterative process is terminated.

${error}_{n+1}=\mathrm{}\frac{\left|\left|{\left\{\mathrm{\Delta }{\epsilon }_t\right\}}_{n+1}\right|\right|}{\left|\left|{\left\{{\epsilon }_t\right\}}_{n+1}\right|\right|}\le tol$ (24)

4 NUMERICAL CODE AND VALIDATIONS

4.1 Numerical code

The CSM algorithm is illustrated in three figures. Figure 4 shows the problem solution procedure using the Newton-Raphson method. Figure 5 presents the constitutive model for the concrete layers. In this model, the behavior of the concrete is evaluated based on its current stage, whether cracked or uncracked. After determining the principal stresses of the concrete layer based on the compressive and tensile constitutive models shown in Figure 1, the layer's stiffness matrix and internal force vector are calculated. These are then added to the shell's corresponding global matrix and internal forces vector.

Figure 4
CSM solution procedure.

Figure 5
Concrete constitutive model.

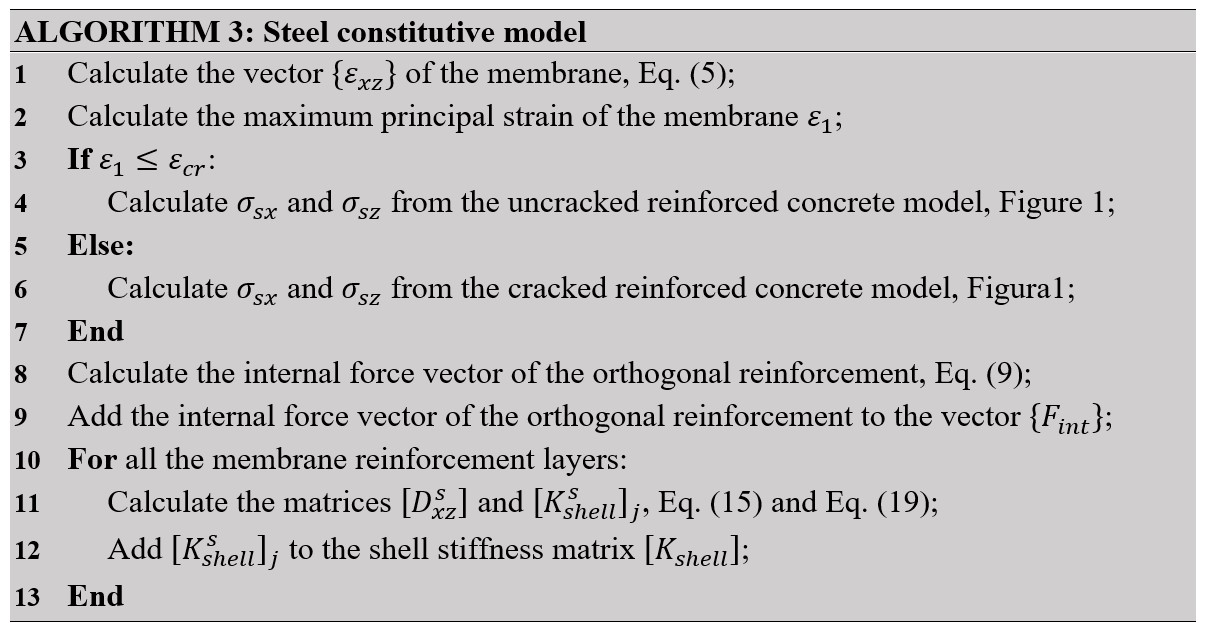
The constitutive model of the orthogonal reinforcement is shown in Figure 6. Stresses in the reinforcement are evaluated using the constitutive model of uncracked reinforced concrete when the fictitious membrane element containing the reinforcement is uncracked, see Figure 1. On the other hand, it is evaluated using the CMM when the element is cracked. For analyzing the load-deformation response of the structure, the CSM solution procedure (Figure 3) is iteratively performed by incrementing the external force vector. This process is repeated until the strain ${\epsilon }_3$ of the outermost layer of the shell reaches the limit value, or until steel reaches ultimate stress.

Figure 6
Steel constitutive model.

4.2 CSM validation

The validation of the CSM was performed by comparing the results with RC panels tested by Polak and Vecchio [²⁰], Lenschow and Sozen [²¹] apud Spathelf [⁶], and Marti et al. [²²]. Aiming the comparison with other numerical methods, these panels were also compared with solutions obtained using the Shell2000 (software developed by Bentz [²³] and which adopts the MCFT to evaluate the behavior of RC membranes), as well as other computational solutions by Spathelf [⁶], Seelhofer [¹⁵], Silva [¹²], Pimentel [⁵], Hrynyk [²⁴] and Luu et al [²⁵].

According to Figures 7a, 8a, 9a and 10a, the panels’ external force vector $\left\{F_{ext}\right\}$ depends on the corresponding vector that defines the shell normalized actions and the load increment factor $t$. These figures also depict the panel characteristics and material properties. To account for the most critical scenario, where reinforcement stresses are highest, the value of $\lambda$ was set to 1 [⁶], indicating maximum crack spacing. As an initial estimate of the strain-curvature vector $\left\{{\epsilon }_t\right\}$, necessary to initialize the solution procedure, Kirchhoff’s thin plate theory was applied, considering linear elastic materials.

Figure 7
SM1 shell analysis results.

Figure 8
SM2 shell analysis results.

Figure 9
B5 shell analysis results.

Figure 10
ML8 shell analysis results.

The RC shells SM1 and SM2, tested by Polak and Vecchio [²⁰], have dimensions to 1524$\times$1524$\times$316 mm. As shown in Figures 7a and 8a, panel SM1 was subjected only to uniaxial bending in the $x$-direction, while panel SM2 was subjected to a compression and tensile plane state and uniaxial bending in the $x$-direction. The tolerance criterion adopted for solving the equations system was 10⁻². This tolerance proved sufficient for experimental validation. The element thickness was discretized into 20 concrete layers. Figures 7b and 8b show the $M_x-{\kappa }_x$ curve obtained experimentally, as well as the predictions from the implemented model (CSM) and other numerical approaches from the literature. As can be seen, the CSM predictions closely match the experimental results, especially in the service load level (reinforcement has not yielded). After yielding, the behavior appears less rigid than the experiment results; however, the ultimate bending moments are similar. The predictions from other numerical solutions were more rigid after concrete cracking compared to the experimental result and the CSM solution. The other approaches presented in Figures 7b and 8b used different models from the one applied in this work to consider tension stiffening, average models such as MCFT and Softened Truss Model (STM) and local models such as F-CMM with fixed and interlocked cracks. These models have different formulations, which can naturally imply different results between the numerical solutions. Therefore, it is up to the user to select the appropriate approach for the case under analysis.

In both panels (SM1 and SM2), processing was stopped when the compressive principal strain of the outermost layer of the shell reached the defined limit, indicating concrete crushing rupture. The average processing time for the analyses was approximately 6 seconds.

In Figures 7 and 8, subfigures (c), (d), and (e) illustrate the concrete layers stresses and reinforcement forces in the section, parallel to the $x-y$ plane, at points A, B, and C, which are indicated on the $M_x-{\kappa }_x$ curve. All points are located in the post-cracking region of the concrete. Point A is close to the beginning of the concrete cracking. Point B before reinforcement yielding and point C after reinforcement yielding. The gray rectangles correspond to the concrete layers, with their lengths depending on the stress value. The red arrows represent the top and bottom reinforcement in the $x$ direction, with their lengths depending on the reinforcement forces. The point in the section where the strain is zero (${\epsilon }_x=0$), the neutral axis, was determined using Equation 2. To better visualize this location, a refined discretization of the section is necessary. As only 20 layers were adopted, the neutral line may intersect a concrete layer with non-null stress. It can be observed that, above the neutral line, the material is in compression. Below it, under tensile, only the reinforcement contributes to the equilibrium of the section. This graphical representation contributes to a better understanding of the behavior of the materials in the respective section. It allows professionals to identify the stresses in the concrete layers, including whether they are cracked or not, and it shows which reinforcements are under tensile and compression.

The B5 reinforced concrete shell, shown in Figure 9, was tested by Lenschow and Sozen [²¹] apud Spathelf [⁶], and has dimensions of 1067$\times$1524$\times$105 mm. According to Figure 9a, the panel has orthogonal reinforcement only on the bottom surface and is subject to biaxial bending (in both directions) and torsion. The authors did not specify the Poisson ratio, so it was assumed to be zero, as observed in Pimentel [⁵]. The remaining missing information was calculated based on the literature review. As a tolerance criterion it was admitted $tol={10}^{-2}$.

The panel was discretized into 100 concrete layers [⁶]. The analyzed curves were $M_x-{\kappa }_p$, $M_x-{\epsilon }_{smx}$, $M_x-{\epsilon }_{smz}$, $M_x-{\epsilon }_{c3}$ and $M_x-{\epsilon }_1$. The term ${\kappa }_p$ represents the bending curvature related to the principal moment, with a direction of 45º relative the $x-z$ axis system. The reinforcement average strains in the $x$ and $z$ directions are indicated by ${\epsilon }_{smx}$ and ${\epsilon }_{smz}$. The concrete principal strains are those of the top layer of the shell. Observing the results, it can be seen that the CSM satisfactorily evaluates the behavior of the panel, highlighting the good correlation of the reinforcement strains in Figures 9c and 9d, and the maximum principal strain in Figure 9f. Comparing with the numerical predictions of Spathelf [⁶] and Shell2000, the CSM demonstrated good results, especially in the curve of maximum principal strain ${\epsilon }_1$. In Figure 9f, it can be seen that approximately between the moments 3kNm/m and 5kNm/m there is a disturbance in the numerical solution of the CSM. Despite this, compared to Shell and Spathelf, the CSM presented a representative prediction in view of the experimental data. The average processing time for analyzes in the CSM was 10 seconds. Considering the layers number adopted (100 layers), this is a relatively low time, demonstrating the efficiency of the proposed routine.

Figure 10 presents the details of the ML8 reinforced concrete shell tested by Marti et al. [²²]. The panel’s dimensions are 1700$\times$1700$\times$200 mm, and it was subjected only to torsional moments (pure torsion). The Poisson ratio for the analysis of this shell was zero [⁵], while the other information not provided was obtained from the literature review. For the solution procedure, a tolerance of 10^-2 was imposed for the convergence criterion. The panel thickness was discretized into 100 concrete layers. The results of the analysis are shown in Figure 10, evaluating the behaviors $M_{xz}-{\kappa }_{xz}$, $M_{xz}-{\epsilon }_{mx}$, e $M_{xz}-{\epsilon }_{mz}$. It can be seen from the figures that the CSM responses show good prediction when compared with the experimental data, especially in Figures 10b and 10d. The shell fails due to concrete crushing under very close to the experiment’s ultimate torsion moments. When compared with the numerical solution of Seelhofer [¹⁵], both present similar behaviors. On the other hand, the Shell2000 prediction was more rigid than the other results. The analysis for the pure torsion state was more costly, with an average processing time of 15 seconds. Pure torsion problems tend to be more complex than pure bending problems. It is believed that this longer time is related to the initial estimative. In the first points of the curve, the code presented difficulty in solution convergence. Even so, the CSM presented adequate results.

Table 2 presents a comparative analysis between the cracking and ultimate moments corresponding to the experiment ($M_r^{exp}$ and $M_u^{exp}$) and those predicted by the CSM ($M_r^{CSM}$ and $M_u^{CSM}$). For the SM and B series panels, these moments correspond to $M_x^{}$, while for the ML8 panel, it is the torsional moment $M_{xz}^{}$. According to statistical data, the mean of the relationships between cracking moments ($M_r^{CSM}$/$M_r^{exp}$) was 0.99, with a coefficient of variation indicating a degree of dispersion of approximately 19.19%. Evaluating the relationship between the ultimate moments ($M_u^{CSM}$/$M_u^{exp}$) in Table 2, it can be seen that the mean was higher (1.05), with a lower degree of dispersion (7.62%). From this relationship, it is observed that the greatest difference between moments was 19%, corresponding to panel B5. Unlike the other panels, panel B5 has reinforcement only on its bottom face. To better investigate the causes of this difference, it is necessary to evaluate experimental data from panels with similar characteristics.

Table 2
Comparative analysis between experimental and numerical results.

Panel	$M_r^{exp}\mathrm{}$kNm/m	$M_u^{exp}\mathrm{}$kNm/m	$M_r^{CSM}\mathrm{}$kNm/m	$M_u^{CSM}\mathrm{}$kNm/m	$M_r^{CSM}/M_r^{exp}$	$M_u^{CSM}/M_u^{exp}$
SM1	75	442.27	54	434	0.72	0.98
SM2	45	297.87	52	309	1.16	1.04
B5	2.43	10.80	2.86	12.89	1.18	1.19
ML8	27.07	63.03	24.39	63	0.90	1.00
Mean					0.99	1.05
Standard deviation					0.19	0.08
Coefficient of variation					19.19%	7.62%

5 CASE STUDY: COMPRESSIVE MEMBRANE ACTION

This section presents the study conducted on the Compressive Membrane Action (CMA) in bridge deck slabs. Using the CSM, the failure load of a specimen simulating a reinforced concrete bridge slab was determined. The numerical result is then compared with the result obtained from the experimental test.

5.1 Compressive membrane action

The Compressive Membrane Action (CMA) is a phenomenon observed in RC slabs with some form of horizontal restraint, resulting in an increase in the strength capacity of these slabs due to the development of compressive stresses. Two conditions are necessary for the development of CMA in reinforced concrete slabs: ​​cracking of the concrete at the location of highest positive moment and the imposition of translational restraint, whether through edge beams, adjacent slabs, or surrounding concrete (Figure 11a). Concrete cracking causes the neutral axis to migrate upward, accompanied by an in-plane expansion of the slab at its boundaries. When contained by some restraint, the displacements arising from this expansion induce the formation of compression membrane forces in the slab plane. This increases the slab strength, both in flexure and punching shear, to values ​​greater than expected [²⁶]. According to Figure 11b, in bridge deck slabs, the CMA develops through the formation of a compressed shell around the location of the tire load application, as described by Csagoly and Lybas [²⁷], [³].

Figure 11
Compressive membrane action.

The CMA effect significantly reduces stresses in the slab reinforcement, allowing for the design of elements with lower reinforcement ratios, which is economically favorable. In bridge deck slabs subjected to significant stress fluctuations, the phenomenon of reinforcement fatigue is present. Therefore, understanding CMA is essential for ensuring both economical and secure design. In research conducted by Brotchie and Holley [²⁸] apud [²⁷], it was observed that CMA considerably mitigates the problem of fatigue in bridge slabs [²⁷]. This discovery prompted revisions in the United State’s bridge design code. Particularly noteworthy is the AASTHO [³] recommendation regarding the Fatigue Limit State, which states that verification is unnecessary for concrete deck slabs of multigirder bridges, as long as the spacing between beam axes does not exceed 4.1 m.

5.2 Investigated structure

For this study, the S2 specimen tested by Hon et al. [²⁶] was utilized. The specimen, depicted in Figure 12, was constructed to simulate a RC bridge slab supported on beams. Initially, the slab is built as a single unit and later divided into three sections by saw cuts made at two locations. Figure 12 illustrates the characteristics of the concrete and steel, providing a detailed cross-section of the slab. Properties of the materials not specified by the author were adopted in accordance with ABNT NBR 6118 [²]. The ultimate stress $f_{su}$ adopted was equal to the integer value immediately higher than the yield stress (due to CMM restrictions [¹¹]).

Figure 12
Specimen details and location of the loading area for each test.

The specimen was subjected to load tests at four different points, of which S2 and S2Fa are considered in this study (see Figure 12). The first test performed was S2, with a monotonic load applied in the middle of the span over an area of 200$\times$100 mm, simulating a vehicle tire. In the S2Fa test, a uniform load was applied over an area of 300$\times$50 mm, causing significant bending along the longest span of the slab. To conduct the tests, the specimen was restrained at all four corners.

5.3 Determination of rupture load via CSM

The specimen was initially analyzed using the SAP2000 structural analysis software to determine internal forces ($M_x^{SAP}$, $M_z^{SAP}$ and $M_{xz}^{SAP}$) under the applied load, employing linear elastic finite elements analysis. The ratios of these forces ($M_z^{SAP}/M_x^{SAP}$ e $M_{xz}^{SAP}/M_x^{SAP}$) were used in subsequent CSM analysis. In SAP2000, the specimen was modeled with frame elements for edge beams and diaphragms, and shell-thin elements for the slabs. The finite element mesh of the slab consists of 864 elements with dimensions of 50×50 mm, 50×32.5 mm, and 50×25 mm. Based on the authors experience, this mesh was considered sufficient for this study. To simulate saw cut and gap, nodes of neighboring elements at these locations were disconnected, creating mesh discontinuities. Given that the ratio of internal forces ($M_z^{SAP}/M_x^{SAP}$ e $M_{xz}^{SAP}/M_x^{SAP}$) in linear analysis is independent of the applied load, we chose an arbitrary value of 10000 kN/m² for the applied load $q_{SAP}$. After analysis, the maximum forces were identified, primarily occurring at the central point of load application. Given the analysis and slab modeling approach, it behaved similarly to a plate, hence the membrane forces were negligible. Figure 13 presents the bending moment diagram in the $z$-direction obtained via SAP2000 for each test. Torsional moments were approximately zero ($M_{xz}^{SAP}/M_x^{SAP}\approx 0$), resulting solely in bending moments in the $x$ and $z$ directions.

Figure 13
Bending moment diagram in $z$ direction.

Based on the results obtained from SAP2000, the external force vectors for the CSM analysis are defined by equations Equation 25 and Equation 26, corresponding to tests S2 and S2Fa. As it is a non-linear analysis, this proportion between forces is not maintained, due to the physical non-linearity of the concrete. However, the methodology presented constitutes only a simplified way of obtaining the internal forces in the structure, using a tool widely used in design offices, for a subsequent non-linear analysis and verification of the relevance of the CMA in the present study.

$\left\{F_{ext}\right\}=\mathrm{}t{\left\{\begin{array}{cccccc}0& 0& 0& 1& 1.2& 0\end{array}\right\}}^T$ (25)

$\left\{F_{ext}\right\}=\mathrm{}t{\left\{\begin{array}{cccccc}0& 0& 0& 1& 5.63& 0\end{array}\right\}}^T$ (26)

For the CSM analysis, the shell was discretized into 100 concrete layers with a convergence criterion set at 10^-2. During the analysis, the vector $\left\{F_{ext}\right\}$ corresponding to shell element failure was evaluated, which occurs when either material reaches its ultimate strength. By applying the ultimate bending moment $M_x^{CSM}$ in equation Equation 27, the failure load $P_u^{CSM}$ can be estimated using the CSM approach. The term $A_{load}$ denotes the load area.

$P_u^{CSM}=q_{SAP}{A}_{load}\frac{M_x^{CSM}}{M_x^{SAP}}$ (27)

5.4 Results and discussions

Table 3 presents the failure loads of the slabs subjected to tests S2 and S2Fa, comparing the experiment results $P_u^{exp}$ with the predictions from the CSM routine $P_u^{CSM}$. According to Hon et al. [²⁶], the slab in test S2 failed in a brittle manner, characterized by a sudden drop in load whereas the slab in test S2Fa failed due to concrete crushing. In both numerical analyses using CSM, the rupture of the bottom reinforcement in the $z$ direction was observed, after the material yielded, as the steel model is approximately an elasto-plastic curve. According to Table 3, for test S2, the estimated failure load by CSM was 70.77 kN, which is approximately 50% lower than the experimental value. For the S2Fa test, the estimated failure load was 46.06 kN, approximately 38% lower than the experimental value.

Table 3
Observed results.

Test	$P_u^{exp}$ [kN]	$P_u^{CSM}$ [kN]	$P_u^{CSM}/P_u^{exp}$ [%]
S2	142.85	70.77	49.5
S2Fa	74.00	46.06	62.2

Despite the good prediction of the CSM compared to the experimental validations presented in section 4, the percentage differences between the failure loads $P_u^{EXP}$ and $P_u^{CSM}$ show in Table 3 are significant. The percentage difference for test S2 (50%) is higher than that for test S2Fa (38%). These observed differences can be attributed, at least in part, to the CMA. The geometry of the S2 test, as it has more surrounding concrete, is more favorable for the development of CMA, compared to the S2Fa test. This explains why $P_u^{EXP}$ for test S2 was significantly higher than for S2Fa. According to this hypothesis, the reason why the CSM failure loads are significantly lower than those in the experiment is that the model is unable to incorporate the CMA. Consequently, the CSM underestimates the failure load of the structure and overestimates the reinforcement stresses. These results are expected. To accurately assess its effects on slab strength, the problem studied here requires analysis using a three-dimensional approach with solid elements to consider the triaxial stress state. Since the CSM is based on Kirchhoff thin plate theory, it fails to meet the necessary conditions mentioned above, thereby unable to capture the CMA. Kirchhoff theory is also incorporated into the shell element used in SAP2000, widely employed in bridge design practice.

The results observed in this study demonstrate that although the CSM showed good results in validations, for certain problems, other aspects need to be considered to model the problem satisfactorily. In the present case, a 3D finite element formulation may be necessary, including constitutive models similar to the CMM and CSM. The development of studies and models capable of incorporating the CMA may have significant impacts on the country's bridge projects. The standards that regulate the design of reinforced concrete bridges, such as ABNT NBR 7187 [²⁹] and ABNT NBR 6118 [²], do not have design criteria that consider the benefits of CMA. This contrasts with AASHTO [³], particularly in the verification of the Fatigue Limit State, where CMA can influence.

6 CONCLUSIONS

This work presents a nonlinear computational routine capable of determining the serviceability stresses and at the rupture of orthogonal reinforcement in cracked reinforced concrete shell elements. For this purpose, the layered method and the membrane model CMM were used. The routine was validated through experimental and numerical results of panels available in the literature. Additionally, a case study on the CMA in concrete bridge deck slabs was conducted from the comparison of experimental results and analysis via CSM. Based on this, it can be concluded that:

1. The computational implementation of CSM has demonstrated its ability to accurately evaluating the behavior of reinforced concrete thin shell elements under various external stresses, consistent with experimental results. It shows good prediction of serviceability behavior, when the reinforcement is not yielded, and ultimate limit state. Compared to numerical solutions, the results obtained using CSM performed well and, in some cases, were superior.

2. In terms of computational cost, based on the criteria set for the iterative-incremental method, the processing time was approximately 15 seconds for elements subjected to pure torsion and an average of 8 seconds for other loading conditions. Considering this time, the proposed routine is appropriate for project office practice. The higher computational cost for elements subjected to pure torsion was primarily due to the initial estimative used for the first iteration, where the algorithm required more time to find an equilibrated configuration. This issue could potentially be mitigated by exploring alternative initial estimative, which could be a focus of future investigations.

3. In the study on compressive membrane action (CMA) in bridge deck slabs, the failure load obtained from the CSM was significantly lower than observed in the tests for both cases. This indicates that while the procedure performed well in validation with experimental data, it was unable to accurately model this structural behavior. As expected, two-dimensional models based on Kirchhoff theory, like the CSM, cannot fully capture the real behavior of slabs subjected to CMA. This phenomenon involves a larger spatial dimension and requires study using three-dimensional models with solid elements.

• Financial support: Postgraduate scholarship awarded by the Pernambuco Science and Technology Support Foundation (FACEPE).
• Data Availability: The data that support the findings of this study are available from the corresponding author, B. C. Fernandes, upon reasonable request.
• How to cite: B.C. Fernandes, J.R.B. Silva, and B. Horowitz, “Nonlinear analysis of reinforced concrete shells and the compressive membrane action on bridge deck slabs,” Rev. IBRACON Estrut. Mater., vol. 18, no. 2, e18201, 2025, https://doi.org/10.1590/S1983-41952025000200001.

REFERENCES FORMATS

• ¹ N. H. Eshwarappa and A. R. Gangolu, “Fatigue behavior of lightly reinforced concrete beams in flexure due to overload,” in Proc. 9th Int. Conf. Fract. Mech. Concr. Concr. Struct., vol. 9, pp. 1–12, 2016.
• ² Associação Brasileira de Normas Técnicas, Projeto de Estruturas de Concreto, ABNT NBR 6118, 2014.
• ³ American Association of State Highway and Transportation Officials, Bridge Design Specifications, 2014.
• ⁴ S. Kvam, “Implementation of the cracked membrane model for crack width predictions in reinforced concrete shell structures,” M.S. thesis, Dept. Struct. Eng., Norwegian Univ. Sci. Technol., Norway, 2018.
• ⁵ M. J. S. Pimentel, “Numerical modelling for safety examination of existing concrete bridges,” Ph.D. dissertation, Fac. Eng., Univ. Porto, Porto, Portugal, 2011.
• ⁶ C. A. Spathelf, “Fatigue performance of orthogonally reinforced concrete slabs,” Ph.D. dissertation, Inst. Struct. Eng., Swiss Fed. Inst. Technol. Zurich, Zurich, 2017.
• ⁷ W. Kaufmann, “Strength and deformations of structural concrete subjected to in-plane shear and normal forces,” Ph.D. dissertation, Inst. Struct. Eng., Swiss Fed. Inst. Technol. Zurich, Zurich, 1998. http://doi.org/10.1007/978-3-0348-7612-4
  » http://doi.org/10.1007/978-3-0348-7612-4
• ⁸ P. Marti, M. Alvarez, W. Kaufmann, and V. Sigrist, "Tension chord model for structural concrete. structural engineering international," Struct. Eng. Int., vol. 8, no. 4, pp. 287–298, 1998. http://doi.org/10.2749/101686698780488875
  » http://doi.org/10.2749/101686698780488875
• ⁹ J. A. Overli and S. I. Sorensen, TKT4222 Concrete Structures 3 Trondheim: NTNU, 2012.
• ¹⁰ B. C. Fernandes, “Procedimento numérico para avaliação das tensões em serviço na armadura ortogonal de cascas de concreto armado,” M.S. thesis, Dept. Civil Eng., Univ. Fed. Pernambuco, Recife, PE, Brazil, 2024.
• ¹¹ B. C. Fernandes, J. R. B. Silva, B. Horowitz, and L. F. A. Bernardo, "Comparative study between local and average constitutive models for reinforced concrete panels," Rev. IBRACON Estrut. Mater., in press.
• ¹² J. R. B. Silva, “Análise de pilares-parede de concreto armado via método dos elementos finitos,” Ph.D. dissertation, Dept. Civil Eng., Univ. Fed. Pernambuco, Recife, PE, 2016.
• ¹³ F. J. Vecchio and M. P. Collins, "The modified compression-field theory for reinforced concrete elements subjected to shear," ACI Struct. J., vol. 83, pp. 219–231, 1986.
• ¹⁴ V. Sigrist, “Zum Verformungsvermögen von Stahlbetonträgern,” Ph.D. dissertation, IBK, Zurich, 1995.
• ¹⁵ H. Seelhofer, “Ebener Spannungszustand im Betonbau: Grundlagen und Anwendungen,” Ph.D. dissertation, Inst. Struct. Eng., Swiss Fed. Inst. Technol. Zurich, Zurich, 2009.
• ¹⁶ F. R. Barrales, “Development of a nonlinear quadrilateral layered membrane element with drilling degrees of freedom and a nonlinear quadrilateral thin flat layered shell element for the modeling of reinforced concrete walls,” Ph.D. dissertation, Fac. USC Grad. Sch., Univ. South. California, California, EUA, 2012.
• ¹⁷ Y. X. Zhang, M. A. Bradford, and R. I. Gilbert, "A layered shear-flexural plate/shell element using timoshenko beam functions for nonlinear analysis of reinforced concrete plates," Finite Elem. Anal. Des., vol. 43, no. 11-12, pp. 888–900, 2007. http://doi.org/10.1016/j.finel.2007.05.002
  » http://doi.org/10.1016/j.finel.2007.05.002
• ¹⁸ V. M. A. Leitão and L. M. S. S. Castro, Análise de Estruturas 1: Apontamentos Sobre Análise de Lajes. Lisboa: Inst. Super. Tec., Univ. Lisboa, 2018.
• ¹⁹ A. Vasilescu, “Analysis of geometrically nonlinear and softening response of thin structures by a new facet shell element,” M.S. thesis, Dept. Civ. Environ. Eng., Fac. Grad. Stud. Res., Carleton Univ., Ottawa, Ontario, 2000. http://doi.org/10.22215/etd/2000-04602
  » http://doi.org/10.22215/etd/2000-04602
• ²⁰ M. A. Polak and F. J. Vecchio, "Reinforced concrete shell elements subjected to bending and membrane loads," ACI Struct. J., vol. 91, pp. 261–268, 1994.
• ²¹ R. J. Lenschow and M. A. Sozen, A Yield Criterion for Reinforced Concrete under Biaxial Moments and Forces (Structural Research Series 311). Illinois: Univ. Illinois, 1966.
• ²² P. Marti, P. Leesti, and W. Khalifa, "Torsion tests on reinforced concrete slab elements," J. Struct. Eng., vol. 113, no. 5, pp. 994–1010, 1987. http://doi.org/10.1061/(ASCE)0733-9445(1987)113:5(994)
  » http://doi.org/10.1061/(ASCE)0733-9445(1987)113:5(994)
• ²³ E. Bentz, Shell2000 Toronto: Univ. Toronto, 2000.
• ²⁴ T. D. Hrynyk, “Behavior and modelling of reinforced concrete slabs and shells under static and dynamic loads,” Ph.D. dissertation, Univ. Toronto, Toronto, 2013.
• ²⁵ C. Luu, Y. Mo, and T. T. Hsu, "Development of CSMM-based shell element for reinforced concrete structures," Eng. Struct., vol. 132, pp. 778–790, 2017. http://doi.org/10.1016/j.engstruct.2016.11.064
  » http://doi.org/10.1016/j.engstruct.2016.11.064
• ²⁶ A. Hon, G. Taplin, and R. S. Al-Mahaidi, "Strength of reinforced concrete bridge decks under compressive membrane action," ACI Struct. J., vol. 102, no. 3, pp. 393–401, 2005.
• ²⁷ P. F. Csagoly and J. M. Lybas, "Advanced design method for concrete bridge deck slabs," ACI Struct. J., vol. 11, no. 5, pp. 53–63, 1989.
• ²⁸ J. F. Brotchie and M. J. Holley, Membrane Action in Slabs (Publication SP-30: Cracking, deflection, and ultimate load of concrete slab systems). Farmington Hills, MI: ACI, 1971, pp. 345–377.
• ²⁹ Associação Brasileira de Normas Técnicas, Projeto de Pontes de Concreto Armado e de Concreto Protendido, ABNT NBR 7187, 2022.

Edited by

• Editors: Mauro Real, Daniel Cardoso.

Data availability

Data Availability: The data that support the findings of this study are available from the corresponding author, B. C. Fernandes, upon reasonable request.

Publication Dates

• Publication in this collection
  20 Jan 2025
• Date of issue
  2025

History

• Received
  23 July 2024
• Reviewed
  19 Oct 2024
• Accepted
  05 Nov 2024