Mathematical models to adjust the parameters of in vitro cumulative gas production of diets containing preserved Gliricidia

Modelos matemáticos para ajuste dos parâmetros de produção cumulativa de gás in vitro de dietas contendo Gliricídia conservada

ABSTRACT:

This study examined the use of the Gompertz, Groot, monomolecular, Richards and two-compartment-logistic mathematical models to investigate the kinetics of in vitro gas production of diets composed of combinations of Gliricidia hay or silage. In addition, the effects of Gliricidia hay or silage inclusion on the in vitro cumulative gas production of these diets were evaluated. Rumen fermentation kinetics were analyzed by the in vitro cumulative gas production methodology. The model parameters were estimated using the Gauss Newton method, with the exception of the Richards model, which was used by Marquardt’s algorithm. Model fit was assessed using the determination coefficient, F test for parameters identity, concordance correlation coefficient, root mean square error of prediction, and decomposition of mean square error of prediction into mean error, systematic bias and random error. The models were compared for accuracy (pairwise mean square error of prediction) and precision (delta Akaike’s information criterion). All model evaluation and comparison statistics were calculated using Model Evaluation System software version 3.2.2. The Groot and Richards models did not differ from each other (P>0.05) and were the most precise and accurate (P<0.05). Therefore, the Groot model was selected due to its better accuracy and precision and easier access to the parameters. The inclusion of Gliricidia silage in the diet resulted in an increase in the time to obtain the maximum rate of degradation and in the time after incubation when half of the asymptotic level was reached. The Groot model is recommended to estimate the average curve. Dietary inclusion of Gliricidia silage alters the gas production curve due to the longer time required for the diet to reach the maximum rate of degradation, this can increase the time the diet remains in rumen and promote a reduction in the consumption.

Key words:
fermentation kinetics; Groot model; hay; non-linear functions; silage

RESUMO:

Objetivou-se avaliar os modelos matemáticos Gompertz, Groot, monomolecular, Richards e logístico bicompartimental para estudar a cinética de produção de gás in vitro de dietas compostas de combinações de feno ou silagem de Gliricídia. Além disso, avaliou-se os efeitos da inclusão de feno ou silagem de Gliricídia sobre a produção cumulativa de gás in vitro destas dietas. A cinética de fermentação ruminal foi avaliada pela metodologia de produção cumulativa de gás in vitro. Os parâmetros dos modelos foram estimados usando o método de Gauss Newton, com exceção do modelo de Richards, que foi usado algoritmo de Marquardt. O ajuste dos modelos foi avaliado por meio do coeficiente de determinação, teste F para a identidade dos parâmetros, coeficiente de correlação e concordância, raiz quadrada do quadrado médio do erro da predição e a decomposição do quadrado médio do erro da predição em erro médio, vício sistemático e erro aleatório. Os modelos foram comparados quanto à acurácia (quadrado médio da predição pareado) e quanto à sua precisão (critério de informação delta de Akaike). Todas as estatísticas de avaliação e comparação dos modelos foram calculadas usando o software Model Evaluation System versão 3.2.2. Os modelos de Groot e Richards não diferiram entre si (P>0.05) e foram os mais precisos e acurados (P<0.05). Portanto, modelo de Groot foi selecionado devido apresentar melhor acurácia e precisão e apresentar maior facilidade na obtenção dos parâmetros. A inclusão da silagem de Gliricídia na dieta, resultou em elevação no tempo para obtenção da máxima taxa de degradação e no tempo após a incubação em que metade do nível assintótico foi atingido. Recomenda-se a utilização do modelo de Groot para estimativa da curva média. A inclusão da silagem de Gliricídia altera a curva de produção de gás devido o maior tempo necessário para que a dieta atingisse a máxima taxa de degradação, isso pode elevar o tempo de permanência da dieta no rúmen e promover redução no consumo.

Palavras-chave:
cinética de fermentação; feno; funções não-lineares; modelo de Groot; silagem

INTRODUCTION:

In tropical regions, forage plants change their growth pattern according to seasonal environmental scenarios, creating periods of high and low forage availability (SBRISSIA et al., 2020). Thus, rational use of forage resources when they are more widely available allows for better dietary planning, in addition to lessening the impacts of climatic fluctuations throughout the year. The preservation of surplus forage in the form of hay and/or silage increases the efficiency of use of the produced forage (HASELMANN et al., 2020).

Gliricidia (Gliricidia sepium) is a leguminous tree with great potential for use in livestock due to its high protein value (MARTINELE et al., 2014; SANTANA et al., 2019). Providing this material in preserved form results in production indices similar to those achieved with supplementation with soy (FERNANDES et al., 2020). Evaluating this ingredient in ruminant diets is an important step to elucidate its benefits in animal feeding as well as reduce the costs of production systems (SANTANA et al., 2020), since protein concentrates are one of the costliest items of the animal diet.

The in vitro cumulative gas production technique is widely used for gravimetric and metabolic assessments of feed stuffs (VELHO et al., 2014; SANTANA et al., 2020). However, it is important to use the most suitable mathematical model to obtain the fermentation parameters and; consequently, the fitting of gas production curves, which can vary depending on the model used (SANTOS et al., 2019). Some researchers have used nonlinear models to study the kinetics of in vitro gas production (FARIAS et al., 2011; VELHO et al., 2014; TEIXEIRA et al., 2016; GOMES et al., 2017), especially the Gompertz, Richards, Groot and monomolecular models. However, the two-compartment logistic model, proposed by PELL & SCHOFIELD (1993), has been widely used to estimate the in vitro gas production of feedstuffs and diets for ruminants (OLIVO et al., 2017; DIAZ et al., 2018; SOUZA et al., 2018; OLIVEIRA et al., 2017; LEAL et al., 2020, SANTANA et al., 2020). Nevertheless, VELHO et al. (2014) warned that a single model should not be used indiscriminately for all types of feed; rather, it is essential that different models be evaluated for each experimental situation.

Incorporating Gliricidia as hay or silage in the traditional way sheep feed based on elephant grass hay, soybean meal and cornmeal can allow a reduction in the quantities of soybean meal in the feed. Added to this, reducing the proportion of expensive soy bran should reduce the cost. Thus, in vitro studies, allow the elaboration of hypotheses about the possible responses of the animals when submitted to these diets.

On this basis, the present study examined the use of the Gompertz, Groot, monomolecular, Richards and two-compartment-logistic mathematical models to elucidate the kinetics of in vitro gas production of diets composed of varied combinations of Gliricidia hay or silage. In addition, the effects of including Gliricidia in the form of hay or silage on the in vitro gas production curve of these diets were evaluated.

MATERIALS AND METHODS:

Data of three diets (Table 1) formulated for sheep with an estimated weight gain of 200 g/day and dry matter intake estimated at 3.5% live weight, according to the NRC (2007), were used. Laboratory analyses were performed at the Laboratories of Animal Nutrition and Rumen Fermentation at the Department of Animal Science (DZO) of the Federal University of Sergipe (UFS), located in Aracaju - SE, Brazil. Methodological details of the making of Gliricidia hay and silage can be reported in SANTANA et al. (2020).

Table 1
Ingredient and nutritional compositions of the experimental diets.

	Control	Gliricidia hay	Gliricidia silage
-----------------------------------------------------------------------Ingredient (% diet DM)------------------------------------------------------------------
Elephant grass hay	55.0	10.0	10.0
Gliricidia hay	-	45.0	-
Gliricidia silage	-	-	45.0
Soybean meal	23.5	10.0	10.0
Ground maize	21.5	35.0	35.0
----------------------------------------------------------------------Nutrient content (% DM)-----------------------------------------------------------------
Organic matter	93.9	94.7	93.9
Crude protein	21.7	18.3	18.3
Neutral detergent fiber	55.2	49.1	47.7
Acid detergent fiber	30.5	27.6	27.5
Hemicellulose	28.2	30.3	27.0
Cellulose	28.6	22.0	21.2
Lignin	2.6	6.6	8.2
Total digestible nutrients	65.2	68.4	68.9

Three different races on three different days were run. For each diet, five (repetitions) samples were incubated using rumen fluid collected from three hair sheep provided with ruminal fistula (THEODOROU et al., 1994). The samples were incubated in glass flasks with a total capacity of 200 mL. The 160-mL incubation solution consisted of 80% (128 mL) buffer-mineral solution and 20% (32 mL) rumen inoculum inserted manually with a graduated syringe into glass vials previously washed and dried in a forced-air oven, in which a 1 g sample of the diets (Table 1) was added.

In addition to the 15 vials containing samples of the diets, another four vials were filled only the inoculum and buffer solution to measure possible pressures that were not related to the diets. The incubation solution was prepared as described by THEODOROU et al. (1994), using cysteine-HCl as a reducing agent (MOULD et al., 2005).

After the incubation solution and samples were added, CO₂ was manually injected for seven seconds into each flask, which was then closed and placed in a forced-air oven at 39 °C during the 48 h of incubation. Data were recorded automatically by the ANKOM RF Gas Production System. The amount of gas produced (mL/100 mg DM) at each time (1, 2, 3, 4, 6, 8, 10, 12, 16, 18, 24, 36 and 48 hours after incubation) was corrected by subtracting the value of gas produced in the un sampled vials of the total gas obtained in the vials containing the samples. Pressure values were corrected for volume using the following equation: y = - 0.772 + 6.087x - 0.382x² (R² = 0.95), where: y is the final gas volume in mL; and x is the gas pressure in kilopascal at the respective times (OLIVEIRA et al., 2020).

The cumulative gas production generated in the 48 h was subjected to five mathematical models (Table 2). The parameters of the different functions can be interpreted biologically as follows: P(t) is the cumulative production at time t. Parameter “A” is the asymptotic gas production (mL of gas/100 mg DM). In the Gompertz and Richards models, parameter “B” represents the time of colonization (h) of the particle (lag); in the Groot model, it is the time after incubation at which half of the asymptotic level was reached (h); and in the monomolecular model, it is the specific gas production rate (mL of gas/h). In the Gompertz and Richards models, parameter “k” is the specific gas production rate (mL of gas/h); in the Groot model, it is an integration constant that determines the sharpness of the curve. Lastly, parameter “m” defines the inflection point of the curve for the Richards model.

Table 2
Models considered in this study to describe the in vitro gas production curve of diets composed of different combinations of Gliricidia hay or silage.

Model	Equation	N of parameters
Gompertz	$P\left(t\right)=\mathit{A}\exp (-B\exp (-\mathit{kt}\left)\right)$	3
Groot	$P\left(t\right)=\mathit{A}/(1+(B^k/t^k)$	3
Monomolecular	$P\left(t\right)=\mathit{A}(1-\exp (-\mathit{Bt}\left)\right)$	2
Richards	$P\left(t\right)={A(1-B\exp (-\mathit{kt}\left)\right)}^m$	4
Two-compartment logistic	$P\left(t\right)=\mathit{vNFC}/(1+\exp (2-4\mathit{kdNFC}(t-L)\left)\right)\mathrm{}+\mathrm{}\mathit{vFC}/(1+\exp (2-4\mathit{kdFC}(t-L)\left)\right)$	5

In the two-compartment logistic model, “vNFC” and “vFC” represent the volume of gas produced from the degradation of non-fibrous and fibrous carbohydrates, respectively; “kdNFC” and “kdFC” are the respective degradation rates of non-fibrous and fibrous carbohydrates; and “L” is the time of colonization (h) of the particle (lag).

The parameters of the Gompertz, Groot, monomolecular and two-compartment logistic models were estimated using the modified Gauss Newton method, by the NLIN procedure of SAS software (SAS University Edition (version 12), Sas Institute Inc. Cary, CA, USA). The maximum number of iterations used was 100. Because of the difficulty in fitting the Richards model by the Gauss Newton method, due to the non-convergence of the iterative process, the Marquardt algorithm was used for fitting iterations, adopting 200 iterations as a maximum number.

The following criteria were used to evaluate the models: determination coefficient (R²) and F test for the identity of the parameters (β0 = 0 and β1 = 1) of the regression of predicted on observed data; concordance correlation coefficient (CCC); root mean square error of prediction (RMSEP); and decomposition of the mean square error of prediction (MSEP) into mean error, systematic bias and random error. The models were compared as to their accuracy by pairwise mean square error of prediction (pMSEP) analysis and for precision by the delta Akaike information criterion (AIC) (TEDESCHI, 2006).

Statistical calculations for the evaluation and comparison of the models were performed using Model Evaluation System version 3.2.2 (http://nutritionmodels.tamu.edu/mes.htm, College Station, Tx, USA; TEDESCHI, 2006). Once the model that best described the average in vitro gas production curve of diets was chosen, the effect of diets on gas production kinetics was evaluated using a dummy variable, which consisted of creating binary variables (0 or 1) to represent and compare experimental treatments, and when one of the treatments received 1, the others received 0 (REGAZZI, 2003).

The variables obtained from the parameters of the chosen model were calculated as described in GROOT et al. (1996), namely, Ti (h): time of inflection of the curve; Tmax (h): time at which the rate of degradation is maximum; and Rmax (mL of gas/h): maximum fractional rate of substrate degradation. The Ti, Tmax and Rmax variables were subjected to analysis of variance by the PROC GLM command and means were compared using Tukey’s test in the SAS statistical package (SAS University Edition (version 12), Sas Institute Inc. Cary, CA, USA). The significance level was set at 5% for all statistical analyses.

RESULTS:

All models showed average cumulative gas production estimates and standard deviation close to the observed data as well as high determination coefficients (above 95%) of the regression of predicted on observed data (Table 3). The Gompertz, Groot, Richards and two-compartment logistics models generated predictions similar (P>0.05) to the observed data (β0 = 0 and β1 = 1), whereas the monomolecular model generated predictions different from the data (P<0.05).

Table 3
Evaluation of the adequacy of models for estimating the in vitro gas production of diets composed of varied combinations of Gliricidia hay or silage.

Model	Mean	SD	R2	P-value	CCC	RMSEP	Decomposition of MSEP (%)
							ME	SB	RE
Gompertz	13.58	8.46	0.978	0.846	0.989	1.26	0.247	0.652	99.101
Groot	13.55	8.54	0.982	0.971	0.991	1.15	0.045	0.117	99.838
Monomolecular	14.08	7.35	0.952	0.001	0.960	2.24	6.227	23.437	70.336
Richards	13.51	8.59	0.981	0.994	0.990	1.18	0.009	0.022	99.970
Two-compartment logistic	13.60	8.44	0.977	0.794	0.988	1.30	0.339	0.904	98.758
Observed data	13.52	8.66	-	-	-	-	-	-	-

• SD = standard deviation; R² = coefficient of determination; P = probability value associated with the F test for the identity of the parameters of the regression of observed on predicted data; CCC = concordance correlation coefficient; RMSEP = root mean square error of prediction; MSEP = mean square error of prediction; ME = mean error; SB = systematic bias; RE = random error.

According to CCC analysis, all models were accurate and precise, as the CCC was greater than 0.95 for all of them, the closer to one the better. The RMSEP analysis revealed that the monomolecular model has a lower power to predict the exact gas production value, with a RMSEP of 2.24 mL of gas/100 mg of DM, whereas the other models showed average RMSEP of almost half of this value.

The decomposition of the MSEP showed that the Gompertz, Groot, Richards and two-compartment logistic models had more than 97% of their deviations attributed to random errors, which does not indicate any mean or systematic deficiency of these models. The monomolecular model, conversely, showed about 23% of the deviations associated with a systematic bias, that is, a multiplicative error in the predicted values. The cumulative gas production curves of the diets as estimated by each model are shown in Figure 1.

Figure 1
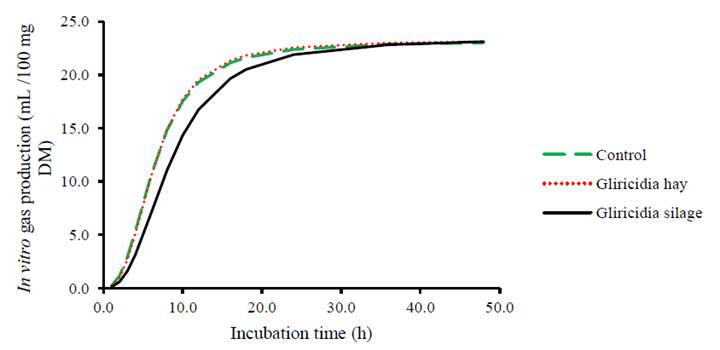
Cumulative production curves of control diet gases (elephant grass hay + soybean meal + corn meal), gliricidia hay treatment (elephant grass hay + soybean meal + corn meal + gliricidia hay) and treatment gliricidia silage (elephant grass hay + soybean meal + cornmeal + gliricidia silage), projected from the parameters estimated by each model.

In the comparison between the models regarding accuracy and precision, the Groot and Richards models did not differ from each other and were more precise (P<0.05) and accurate (P<0.05) than the other models. Thus, either one can be used to estimate the in vitro cumulative gas production curve of diets composed of different combinations of Gliricidia hay or silage.

However, the Groot model was selected due to its better accuracy and precision and easier access to the parameters. Like this, according to the Groot model, there was no difference (P>0.05) between the curves of the control diet and the diet containing Gliricidia hay (Table 4 and Figure 2). However, the inclusion of Gliricidia silage in the diet changed the fermentation kinetics, which generated a cumulative gas production curve different from that obtained with other experimental diets (Table 4 and Figure 2).

Table 4
Equations and variables obtained by the Groot model of in vitro gas production of diets composed of varied combinations of Gliricidia hay or silage.

Diet	Equation		Variable1
			Ti	Tmax	Rmax
Control	$22.9679/\left(1+\left({6.2445}^{2.5507}/t^{2.5507}\right)\right)$		6.01	7.47a	0.213
Gliricidia hay	$23.2054/\left(1+\left({6.4178}^{2.6524}/t^{2.6524}\right)\right)$		6.61	7.77a	0.216
Gliricidia silage	$23.1852/{\left(1+\left({8.1905}^{2.2658}/t^{2.2658}\right)\right)}^{*}$		6.12	9.08b	0.140
SEM		0.512		0.31	0.022
P-value		0.695		0.012	0.061

• ^*= (p<0.05). Means followed by different letters differ statistically by Tukey’s test at 5% significance. ¹Variables obtained from the parameters; Ti (h): time of curve inflection; Tmax (h): time at which degradation rate is maximum; Rmax (mL of gas/h): maximum fractional rate of substrate degradation.

Figure 2
Cumulative production curves of control diet gases (elephant grass hay + soybean meal + corn meal), gliricidia hay treatment (elephant grass hay + soybean meal + corn meal + gliricidia hay) and treatment gliricidia silage (elephant grass hay + soybean meal + cornmeal + gliricidia silage).

There was no effect of diet (P>0.05) on the time of inflection of the curve or the maximum fractional rate of degradation (Table 4). However, the diet containing Gliricidia in the form of silage took longer (P<0.05) to reach the maximum degradation rate.

DISCUSSION:

With the exception of the monomolecular model, all other models showed minimal differences in the model adequacy evaluation criteria. Thus, according to the adopted evaluation criteria, the Gompertz, Groot, Richards and two-compartment-logistic functions would have similar fits. In this way, the sole evaluation of the models, as routinely done (VELHO et al., 2014; FARIAS et al., 2011; GOMES et al., 2017), without a comparison in terms of accuracy and precision, may not be a good option.

The literature describes several variations in the models for the fitting of the in vitro cumulative gas production curve of ruminant diets. FARIAS et al. (2011) studied mathematical models to evaluate gas production from babassu (Orbignya speciosa) meal and cake and preferred the two-compartment logistic model. VELHO et al. (2014) chose the Gompertz model to describe the kinetics of in vitro gas production of maize silages. TEIXEIRA et al. (2016) investigated the kinetic parameters of rumen degradation of protein concentrates (soybean meal and cotton cake) and recommended the Richards model.

This divergence regarding the different adjusted models is theoretically understandable, as it depends on the fermentation pattern of the feedstuffs under study. However, those authors (VELHO et al., 2014; FARIAS et al., 2011; TEIXEIRA et al., 2016) selected the models based on evaluation criteria (root mean square error of prediction, R², mean square error, among others) without comparisons to determine whether the differences in these criteria are indeed significant.

In the present study, the models were compared for accuracy and precision, as suggested by TEDESCHI (2006), and the Groot and Richards functions were found to be more precise and accurate than the others, which indicates that both can be used to estimate the in vitro cumulative gas production curve of diets. However, despite the good fit, the Richards model showed convergence problems in the iterative process, requiring the use of the Marquardt algorithm and an increase in the number of iterations, possibly because this model needs to estimate an additional parameter. Other authors have also reported convergence difficulties using the Richards model (ZWIETERING et al., 1990; KOPUZLU et al., 2014).

In addition, a model with three parameters-as is the case with the Groot model-will exhibit more degrees of freedom in the estimates, which can be important when a curve displays a smaller amount of information (ZWIETERING et al., 1990). It is also important that all parameters have biological significance. In this respect, the Groot model has advantages over the other functions in that it does not assume a constant fractional rate of fermentation (GROOT et al., 1996).

The kinetics of cumulative gas production depends on a sequence of processes. Immediately after incubation, the feed is partially solubilized (ÍTAVO et al., 2016) and compounds with greater solubility are quickly fermented (COSTA et al., 2011; RIBEIRO et al., 2011). Subsequently, the less soluble parts start to ferment (SILVA et al., 2017). In this way, the fractional rate decreases exponentially throughout the incubation time due to the use of substrate by the ruminal microbiota (PELL & SCHOFIELD, 1993). Therefore, models that consider a constant fractional rate do not provide an accurate simulation of the phenomena that occur in the rumen environment. For these reasons, we chose the Groot model to fit the in vitro cumulative gas production curve of the diets.

The similarity between the curves of the control and Gliricidia hay diets fitted by the Groot model may have been due to the greater participation of maize in the diets containing Gliricidia. The inclusion of Gliricidia allowed for a reduction in the proportion of soybean, whereas the proportion of maize was increased, thereby increasing the supply of soluble carbohydrates and rapidly fermentable carbohydrates such as starch for the microbial population.

In contrast, the inclusion of Gliricidia silage generated a different curve from those obtained with the other diets, especially for parameter “k”, which determines the sharpness of the curve. This may have been a reflection of the reduction in soluble carbohydrates during the ensiling process, because these carbohydrates are used as substrate for lactic fermentation in the silo (ZARDIN et al., 2017; SANTANA et al., 2019). Coupled with this is the higher concentration of lignin and cellulose in the diet with Gliricidia silage.

The higher concentration of lignin and cellulose in the diet with Gliricidia silage also explains the longer time taken to obtain the maximum rate of substrate degradation, in that diet. Lignin works as a mechanical barrier against the action of rumen microorganisms (DÍAZ et al., 2018), which can increase the time spent by microorganisms to colonize the particle (OLIVEIRA et al., 2017) and; consequently, the time for microorganisms to reach maximum activity.

These findings may be important for sheep production systems. It is possible to partially replace soybean meal with Gliricidia hay in lamb feeding without impairing ruminal fermentation kinetics. However, additional studies with sheep (in vivo) to determine fed intake and animal performance on these or similar feeds are needed to confirm whether these laboratory (in vitro) findings can be reflected in improved production. Other shrub legumes it can also be used depending on availability.

CONCLUSION:

The Groot and Richards models best describe the kinetics of in vitro gas production of diets with Gliricidia hay or silage. However, the Groot model is recommended to estimate the average curve due to its ease in obtaining the parameters and providing biological explanation. The inclusion of Gliricidia silage alters the fermentation kinetics of the diet. Therefore, it is recommended to use Gliricidia preserved in the form of hay.

ACKNOWLEDGEMENTS

The Coordenação de Aperfeiçoamento de Pessoal de Nível Superior - Brasil (CAPES) - Financing Code 001 and the National Council funded this research for Conselho Nacional de Desenvolvimento Científico e Tecnológico (CNPq). Supported by the Universidade Federal de Sergipe and Universidade Federal de Mato Grosso do Sul.

REFERENCES

• COSTA, V. A. C. et al. Intake and rumen dynamics of neutral detergent fiber in grazing cattle supplemented with non-protein nitrogen and, or true protein during the rainy season. Revista Brasileira de Zootecnia, v.40, n.12, p.2805-2814, 2011. Available from: <Available from: https://doi.org/10.1590/S1516-35982011001200027 >. Accessed: May. 20, 2020. doi: 10.1590/S1516-35982011001200027.
  » https://doi.org/10.1590/S1516-35982011001200027.» https://doi.org/10.1590/S1516-35982011001200027
• DÍAZ, T. G. et al. In vitro gas production kinetics and digestibility in ruminant diets with diferente levels of cashew nut shell liquid. Semina: Ciências Agrárias, v.39, n.4, p.1669-1682, 2018. Available from: <Available from: https://doi.org/10.5433/1679-0359.2018v39n4p1669 >. Accessed: Apr. 25, 2020. doi: 10.5433/1679-0359.2018v39n4p1669.
  » https://doi.org/10.5433/1679-0359.2018v39n4p1669.» https://doi.org/10.5433/1679-0359.2018v39n4p1669
• FARIAS, L. N., V. R. et al. Avaliation of bicompartimental logistic and Gompertz mathematical models to estimate gas production from babassu (Orbignya martiana) meal and pie using the semi-automated in vitro technique. Arquivo Brasileiro de Medicina Veterinária e Zootecnia, v.63, n.1, p.136-142, 2011. Available from: <Available from: https://doi.org/10.1590/S0102-09352011000100021 >. Accessed: Oct. 10, 2020. doi: 10.1590/S0102-09352011000100021.
  » https://doi.org/10.1590/S0102-09352011000100021.» https://doi.org/10.1590/S0102-09352011000100021
• FERNANDES, L. S. et al. Performance of sheep grazing Panicum maximum cv. Massai and supplemented with protein sources during the dry season. South African Journal of Animal Science, v.50, n.1, p.1-8, 2020. Available from: <Available from: http://dx.doi.org/10.4314/sajas.v50i1.1 >. Accessed: May. 21, 2020. doi: 10.4314/sajas.v50i1.1.
  » https://doi.org/10.4314/sajas.v50i1.1.» http://dx.doi.org/10.4314/sajas.v50i1.1
• GOMES, M. F. F. et al. In vitro fermentation characteristics of ruminant diets using ethanol extract of brown propolis as a nutritional additive. Revista Brasileira de Zootecnia , v.46, n. 7, p.599-605, 2017. Available from: <Available from: http://dx.doi.org/10.1590/S1806-92902017000700007 >. Accessed: May. 25, 2020. doi: 10.1590/S1806-92902017000700007.
  » https://doi.org/10.1590/S1806-92902017000700007.» http://dx.doi.org/10.1590/S1806-92902017000700007
• GROOT, J. C. J. et al. Multiphasic analysis of gas production kinetics for in vitro fermentation of ruminant feeds. Animal Feed Science and Technology, v.64, n.1, p.77-89, 1996. Available from: <Available from: https://doi.org/10.1016/S0377-8401(96)01012-7 >. Accessed: May. 30, 2020. doi: 10.1016/S0377-8401(96)01012-7.
  » https://doi.org/10.1016/S0377-8401(96)01012-7.» https://doi.org/10.1016/S0377-8401(96)01012-7
• HASELMANN, A. et al. Comparing the effects of silage and hay from similar parent grass forages on organic dairy cows’ feeding behavior, feed intake and performance. Animal Feed Science and Technology , v.267, n.1 p.114560, 2020. Available from: <Available from: https://doi.org/10.1016/j.anifeedsci.2020.114560 >. Accessed: Oct. 10, 2020. doi: 10.1016/j.anifeedsci.2020.114560.
  » https://doi.org/10.1016/j.anifeedsci.2020.114560.» https://doi.org/10.1016/j.anifeedsci.2020.114560
• ÍTAVO, L. C. V. et al. Combinations of non-protein nitrogen sources in supplements for Nellore steers grazing. Revista Brasileira de Saúde e Produção Animal, v.17, n.3, p.448-460, 2016. Available from: <Available from: http://dx.doi.org/10.1590/S1519-99402016000300011 >. Accessed: Jul. 3, 2020. doi: 10.1590/S1519-99402016000300011.
  » https://doi.org/10.1590/S1519-99402016000300011.» http://dx.doi.org/10.1590/S1519-99402016000300011
• KOPUZLU, S. et al. Estimation of growth curve characteristics of Hemsin male and female sheep. Journal of Applied Animal Research, v.42, n.2 p.228-232, 2014. Available from: <Available from: https://doi.org/10.1080/09712119.2013.842479 >. Accessed: Jul. 8, 2020. doi: 10.1080/09712119.2013.842479.
  » https://doi.org/10.1080/09712119.2013.842479.» https://doi.org/10.1080/09712119.2013.842479
• LEAL, E. S. et al. Influence of protodioscin content on digestibility and in vitro degradation kinetics in Urochloa brizantha cultivars. Crop and Pasture Science, v.71, n.3, p.278-284, 2020. Available from: <Available from: https://doi.org/10.1071/CP18357 >. Accessed: May. 25, 2020. doi: 10.1071/CP18357.
  » https://doi.org/10.1071/CP18357.» https://doi.org/10.1071/CP18357
• MARTINELE, I. et al. Abundance and diversity of rumen protozoa in lambs fed Gliricidia sepium silage. Revista Brasileira de Zootecnia , v.43, n.8, p.436-439, 2014. Available from: <Available from: http://dx.doi.org/10.1590/S1516-35982014000800006 >. Accessed: May. 15, 2020. doi: 10.1590/S1516-35982014000800006.
  » https://doi.org/10.1590/S1516-35982014000800006.» http://dx.doi.org/10.1590/S1516-35982014000800006
• MOULD, F. L. et al. 2005. A review and simplification of the in vitro incubation medium. Animal Feed Science and Technology , v.123-124, n.1 p.55-172, 2005. Available from: <Available from: https://doi.org/10.1016/j.anifeedsci.2005.05.002 >. Accessed: Jan. 05, 2020. doi: 10.1016/j.anifeedsci.2005.05.002.
  » https://doi.org/10.1016/j.anifeedsci.2005.05.002.» https://doi.org/10.1016/j.anifeedsci.2005.05.002
• NRC. Nutrient requirements of small ruminants. 2ª ed. Washinton: DC. National Academy of Sciences, 2007. 362p.
• OLIVEIRA, V. S. et al. Regression equation to estimate volume of ruminal gases and correlation between chemical composition and fermentation parameters. Diversitas Journal, v.5, n, 4, p. 3238-3249, 2020. Available from: <Available from: hhttps://doi.org/10.17648/diversitas-journal-v5i4-1205 >. Accessed: Apr. Jan. 14, 2020. doi: 10.17648/diversitas-journal-v5i4-1205.
  » https://doi.org/10.17648/diversitas-journal-v5i4-1205.» hhttps://doi.org/10.17648/diversitas-journal-v5i4-1205
• OLIVEIRA, V. S. et al. Ruminal kinetics of tropical forrages submitted or not to irrigation. Boletim de Indústria Animal, v.74, n.3, p.195-204, 2017. Available from: <Available from: hhttps://doi.org/10.17523/bia.v74n3p195 >. Accessed: Apr. 10, 2020. doi:10.17523/bia.v74n3p195.
  » https://doi.org/10.17523/bia.v74n3p195» hhttps://doi.org/10.17523/bia.v74n3p195
• OLIVO, P. M. et al. Assessing the nutritional value of agroindustrial co-products and feed through chemical composition, in vitro digestibility, and gas production technique. Acta Scientiarum. Animal Sciences, v.39, n.3, p.289-295, 2017. Available from: <Available from: https://doi.org/10.4025/actascianimsci.v39i3.34024 >. Accessed: Oct. 15, 2020. doi: 10.4025/actascianimsci.v39i3.34024.
  » https://doi.org/10.4025/actascianimsci.v39i3.34024.» https://doi.org/10.4025/actascianimsci.v39i3.34024
• PELL, A.; SCHOFIELD, P. Computerized monitoring of gas production to measure forage digestion in vitro. Journal of Dairy Science, v.76, n.4, p.1063-1073, 1993. Available from: <Available from: https://doi.org/10.3168/jds.S0022-0302(93)77435-4 >. Accessed: Oct. 13, 2020. doi: 10.3168/jds.S0022-0302(93)77435-4.
  » https://doi.org/10.3168/jds.S0022-0302(93)77435-4.» https://doi.org/10.3168/jds.S0022-0302(93)77435-4
• REGAZZI, A. J. Test for parameter equality in nonlinear regression models. Revista Ceres, v.50, n.287, p.9-26, 2003. Available from: <Available from: http://www.ceres.ufv.br/ojs/index.php/ceres/article/view/2854/710 >. Accessed: Nov. 10, 2020.
  » http://www.ceres.ufv.br/ojs/index.php/ceres/article/view/2854/710
• RIBEIRO, S. S. et al. Effects of ruminal infusion of a slow-release polymercoated urea or conventional urea on apparent nutrient digestibility, in situ degradability, and rumen parameters in cattle fed low-quality hay. Animal Feed Science and Technology , v.164, n.1-2, p.53- 61, 2011. Available from: <Available from: https://doi.org/10.1016/j.anifeedsci.2010.12.003 >. Accessed: May. 10, 2020. doi: 10.1016/j.anifeedsci.2010.12.003.
  » https://doi.org/10.1016/j.anifeedsci.2010.12.003.» https://doi.org/10.1016/j.anifeedsci.2010.12.003
• SANTANA, J. C. S. et al. In vitro digestion characteristics of various combinations of elephant grass hay, Gliricidia hay or silage, soybean meal and corn meal in rations for sheep. Tropical Grasslands-Forrajes Tropicales. 8, n.2, p.147-152, 2020. Available from: <Available from: http://dx.doi.org/10.17138/TGFT(8)147-152 >. Accessed: Jul. 05, 2020. doi: 10.17138/TGFT(8)147-152.
  » https://doi.org/10.17138/TGFT(8)147-152.» http://dx.doi.org/10.17138/TGFT(8)147-152
• SANTANA, J. C. S. et al. Fermentation characteristics, chemical composition and protein fractioning of Gliricídia silage submitted to different fermentation periods. Boletim de Indústria Animal , 76, n.1, p.1-9, 2019. Available from: <Available from: https://doi.org/10.17523/bia.2019.v76.e1436 >. Accessed: Jul. 05, 2020. doi: 10.17523/bia.2019.v76.e1436.
  » https://doi.org/10.17523/bia.2019.v76.e1436.» https://doi.org/10.17523/bia.2019.v76.e1436
• SANTOS, A. L. P. et al. Generation of models from existing models composition: An application to agrarian sciences. Plos One, v.14, n.1, p.e0214778, 2019. Available from: <Available from: https://doi.org/10.1371/journal.pone.0214778 >. Accessed: Jul. 10, 2020. doi: 10.1371/journal.pone.0214778.
  » https://doi.org/10.1371/journal.pone.0214778.» https://doi.org/10.1371/journal.pone.0214778
• SBRISSIA, A. F. et al. Unravelling the relationship between a seasonal environment and the dynamics of forage growth in grazed swards. Journal Agronomy and Crop Science, v.206, n.5, p. 630-639, 2020. Available from: <Available from: https://doi.org/10.1111/jac.12402 >. Accessed: Nov. 10, 2020. doi: 10.1111/jac.12402.
  » https://doi.org/10.1111/jac.12402.» https://doi.org/10.1111/jac.12402
• SILVA, R. N. P. et al. Ruminal degradability of shell of pods of the lima bean (“Phaseolus lunatus” L.) ammoniated with urea. Revista Brasileira de Saúde e Produção Animal , 18, n.1, p.26-37, 2017. Available from: <Available from: http://dx.doi.org/10.1590/S1519-99402017000100004 >. Accessed: Oct. 12, 2020. doi: 10.1590/S1519-99402017000100004.
  » https://doi.org/10.1590/S1519-99402017000100004.» http://dx.doi.org/10.1590/S1519-99402017000100004
• SOUZA, A. D. V. et al. Thermal decomposition, chemical composition, in vitro digestibility and gas production and in situ degradability of oilseed residues from the biofuel industry. Animal Science Journal, v.89, n.1, p.79-87, 2018. Available from: <Available from: https://doi.org/10.1111/asj.12889 >. Accessed: Sep. 09, 2020. doi: 10.1111/asj.12889.
  » https://doi.org/10.1111/asj.12889.» https://doi.org/10.1111/asj.12889
• TEDESCHI, L.O. Assessment of the adequacy of mathematical models. Agricultural Systems, v.89, n.2-3, p.225-247, 2006. Available from: <Available from: https://doi.org/10.1016/j.agsy.2005.11.004 >. Accessed: Sep. 15, 2019. doi: 10.1016/j.agsy.2005.11.004.
  » https://doi.org/10.1016/j.agsy.2005.11.004.» https://doi.org/10.1016/j.agsy.2005.11.004
• TEIXEIRA, U. H. G. et al. Mathematical models for estimating the parameters of ruminal degradation kinetics of protein concentrates. Revista Brasileira de Saúde e Produção Animal , v.17, n.1, p.73-85, 2016. Available from: <Available from: http://dx.doi.org/10.1590/S1519-99402016000100008 >. Accessed: Nov. 09, 2020. doi: 10.1590/S1519-99402016000100008.
  » https://doi.org/10.1590/S1519-99402016000100008.» http://dx.doi.org/10.1590/S1519-99402016000100008
• THEODOROU, M. K. et al. A simple gas production method using a pressure transducer to determine the fermentation kinetics of ruminant feeds. Animal Feed Science and Technology , v.48, v.3-4, p.185-197, 1994. Available from: <Available from: https://doi.org/10.1016/0377-8401(94)90171-6 >. Accessed: Sep. 24, 2020. doi: 10.1016/0377-8401(94)90171-6.
  » https://doi.org/10.1016/0377-8401(94)90171-6.» https://doi.org/10.1016/0377-8401(94)90171-6
• VELHO, J. P. et al. Mathematical models for adjustment of in vitro gas production at different incubation times and kinetics of corn silages. Semina: Ciências Agrárias , v.35, n.4, p.2531-2540, 2014. Available from: <Available from: https://doi.org/10.5433/1679-0359.2014v35n4Supl1p253 >. Accessed: Sep. 30, 2019. doi: 10.5433/1679-0359.2014v35n4Supl1p253.
  » https://doi.org/10.5433/1679-0359.2014v35n4Supl1p253.» https://doi.org/10.5433/1679-0359.2014v35n4Supl1p253
• ZARDIN, P. B. et al. Chemical composition of corn silage produced by scientific studies in Brazil - A meta-analysis. Semina: Ciências Agrárias , v.38, n.1; p.503-512, 2017. Available from: <Available from: https://doi.org/10.5433/1679-0359.2017v38n1p503 >. Accessed: Sep. 23, 2019. doi: 10.5433/1679-0359.2017v38n1p503.
  » https://doi.org/10.5433/1679-0359.2017v38n1p503.» https://doi.org/10.5433/1679-0359.2017v38n1p503
• ZWIETERING, M. H. et al. Modeling of the Bacterial Growth Curve. Applied and Environmental Microbiology, v.56, n.6, p.1875-1881, 1990. Available from: <Available from: https://www.ncbi.nlm.nih.gov/pmc/articles/PMC184525/ >. Accessed: May. 10, 2019.
  » https://www.ncbi.nlm.nih.gov/pmc/articles/PMC184525/

• CR-2020-0993.R2

Publication Dates

• Publication in this collection
  07 July 2021
• Date of issue
  2021

History

• Received
  10 Nov 2020
• Accepted
  29 Jan 2021
• Reviewed
  07 May 2021