Information theory applications in neuroscienceAbstract
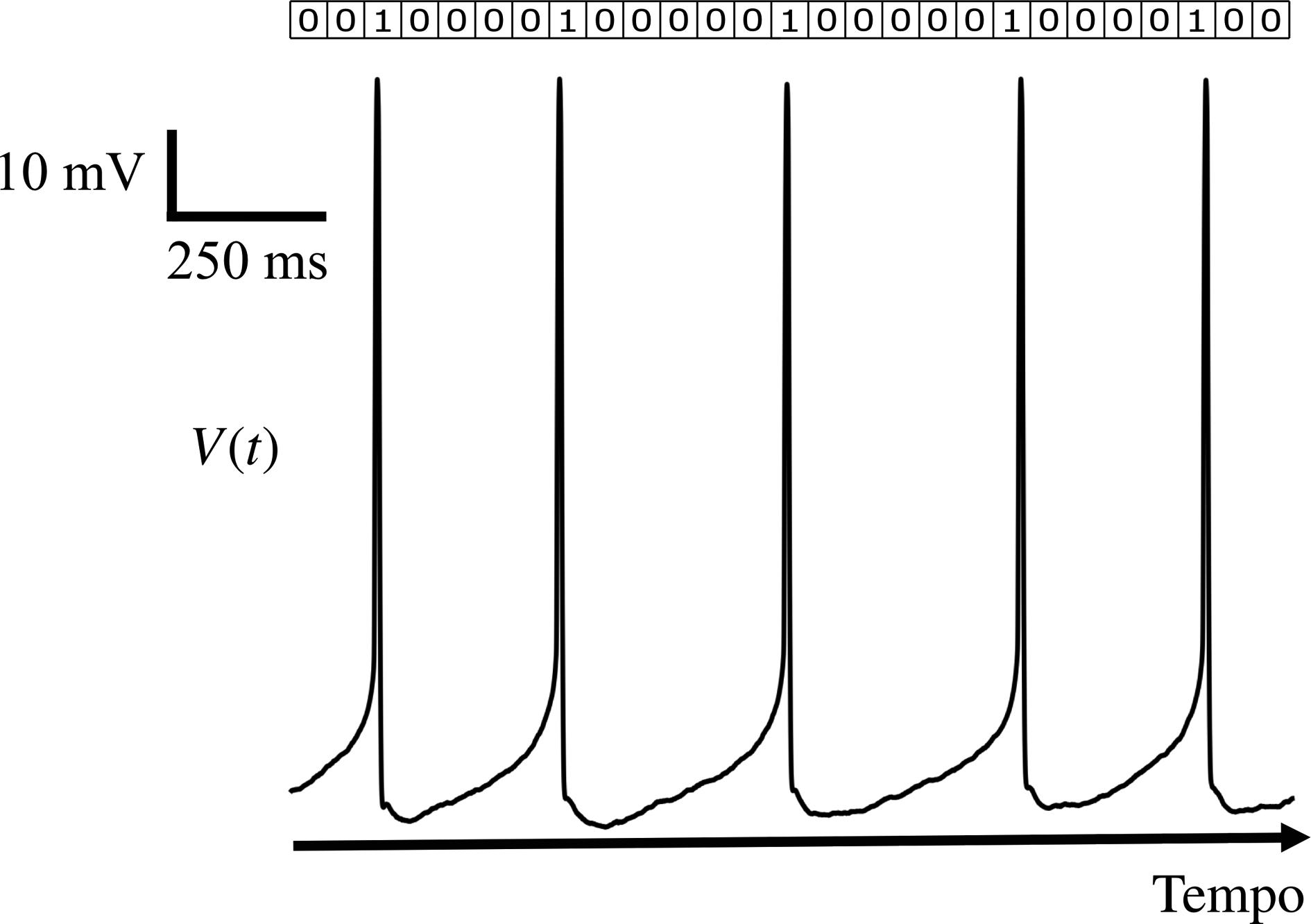
Neurons respond to external stimuli by emitting sequences of action potentials (spike trains). In this way, one can say that the spike train is the neuronal response to an input stimulus. Action potentials are “all-or-none” phenomena, which means that a spike train can be represented by a sequence of zeros and ones. In the context of information theory, one can then ask: how much information about a given stimulus the spike train conveys? Or rather, what aspects of the stimulus are encoded by the neuronal response? In this article, an introduction to information theory is presented which consists of historical aspects, fundamental concepts of the theory, and applications to neuroscience. The connection to neuroscience is made with the use of demonstrations and discussions of different methods of the theory of information. Examples are given through computer simulations of two neuron models, the Poisson neuron and the integrate-and-fire neuron, and a cellular automata network model. In the latter case, it is shown how one can use information theory measures to retrieve the connectivity matrix of a network. All codes used in the simulations were made publicly available at the GitHub platform and are accessible trough the url: github.com/ViniciusLima94/ticodigoneural.
Keywords: information theory; neuroscience; computer simulation; neurons
